Observations on marching Roman legionaries: velocities, energy expenditure, column formations and distances.
Steve Kaye, October 2013.
Contents
- Introduction
- Modern era and Roman march velocities
- An example of a legionary day: schedule, parameters and method
- Energy expenditure and on-road marching velocity of Roman legionaries
- The off-road marching velocity of Roman legionaries
- Roman army multi-column marching
- Summary
- Appendix: The building of temporary marching camps
- Bibliography
- Copyright
Introduction
A combination of modern-day medical and military studies, together with some simple facts about the lives of Roman legionaries, allows: an examination of energy expenditure (EE) and other key physiological factors such as food and water consumption; the legionary rate of march and subsequent implications for the form of marching armies; and insights into the building of temporary marching camps.
Other interesting snippets will be derived and described which in combination with the above, will place the Roman legionary of the early Imperial period, 1st and 2nd centuries AD, more firmly within the bounds ruled by the findings of modern physiological studies.
A key aim of this essay is to identify the typical, day-to-day, march velocity of a Roman army unit as it travelled along roads between marching camps and forts within its province, and also when journeying far greater distances between provinces. Efforts are also made to understand the probable march velocities and column formations for Roman units marching off-road. Not discussed, however, are extraordinary feats of marching, mentioned in some ancient historical accounts.
Note 1: the time format used is hour:min, e.g. '10:13 h' is 10 hours and 13 minutes. Also, a continuous clock is used, so that, times that are actually 'Day 2, 01:30AM' will show as '25:30 h'.
Note 2: when discussing the marching velocities of Roman armed men there is little difference between those for a legionary or auxiliary. Therefore, the term 'soldier' is used to mean either legionary or auxiliary. In addition, where the term 'legion' is used, in relation to the size of a unit, the composition can be of either legionaries or auxiliaries, i.e. a legion of soldiers.
Note 3: much of the discussion derives from a number of spreadsheets that would allow the display of far more numbers than have actually been deployed in this, already dense, essay. For brevities sake, some numbers discussed are not displayed in tables or figures, in which case a note - 'not shown in tables or figures', or similar – appears in parenthesis.
Modern era and Roman march velocities.
This essay examines the work-rates, energy expenditure (EE) and march velocities for soldiers, both modern and Roman, operating over many hours and for many days. With regard to march velocities we are not concerned with short bursts of activity, extraordinary feats of marching (the literature is full of these) or the rather fanciful march rates claimed by some 18th and 19th century units. The main method of investigation is to apply the findings of modern science and medicine to discover the likely performance parameters of Roman soldiers. These data on modern soldiers concentrate on realistic, sustainable, scientifically derived, march velocities for units which were, preferably, operating in the field (not marching out of, and back into, a barrack).
Seeking stipulated, or required, march velocities for modern armies (20th and 21st centuries) does not produce a large number of figures. The US Army and Marines has a standard on-road, march velocity of 2.5 mph (4.0234 kph or 1.1176 m/s), while the British Army supposedly has a standard on-road, march velocity of 3 mph (4.8280 kph or 1.3411 m/s); but there is confusion over whether this last figure is a velocity or the number of miles to be covered in one hour.
The distinction is important because under most conditions marching soldiers are rested for part of the hour (often 10 minutes and the default value used in this essay). Hence, a velocity of 3 mph means that the distance covered in one hour is less than 3 miles; however, if the stated 3 mph is actually the number of miles to be covered in that hour, as mentioned by some sources for the British Army, then assuming a 10 minute rest, the velocity of march is 3.6 mph – a significant increase. In this essay for sustainability reasons, as will be discussed, the British Army 3 mph is classified as a velocity.
Finding modern, official, velocities for off-road marching is even more difficult, with only the US Army and Marines mentioning a figure of 1.5 mph (2.4140 kph or 0.6706 m/s). It is a generalised figure, with no mention of variations in terrain, slope and load carried; factors that will be examined and found to be crucial in determining plausible march velocities.
Having found modern velocities it is necessary, for reasons of comparison, to choose a figure from the many in the literature for a Roman legionary: it is, 2.85 mph (4.5866 kph or 1.2741 m/s) (Whipp, 1998). This is a velocity for marching along Roman roads (typically akin to modern dirt roads, at least as far as the marching surface is concerned), and is bracketed by the US Army 2.5 mph and the British Army 3.0 mph.
No
reliable velocity figures exist for off-road marching by the Roman
legionaries. Some extraordinary figures exist in the ancient
accounts, and some modern authors (Peddie, 1994, p.74) have assigned
a velocity of 3 miles in the hour, but as will be demonstrated, these
values are highly unlikely to have pertained during normal operations
and conditions.
|
MPH |
kph |
Metres/second |
|
1 |
1.6093 |
0.4470 |
|
1.5 |
2.4140 |
0.6706 |
|
2 |
3.2187 |
0.8941 |
|
2.5 |
4.0234 |
1.1176 |
|
2.85 |
4.5866 |
1.2741 |
|
4 |
4.8280 |
1.3411 |
|
3.5 |
5.6327 |
1.5647 |
|
4.0 |
6.4374 |
1.7882 |
Table 1: Conversions between common march velocities. The British Army seems to have an on-road velocity of 3 mph, while for the US Army and Marines it is 2.5 mph. The Roman on-road velocity has been calculated to have been 2.85 mph and 3.4 mph at the quick step (Whip, 1998). The only stated off-road velocity the author can find is that for the US Army at 1.5 mph.
Note: Peddie's “3 miles in the hour” figure, referenced above, has the word 'generous' preceding it, as if he was acknowledging that the velocity is too high. However, he is describing Julius Caesar's battle with the Nervii on the Sambre in 57BC (also known as the Battle of Sabis), when exceptional march velocities might be expected.
An example of a legionary day: schedule, parameters and method.
This section is devoted to describing an example of a legionary day and the variables that have been used in the calculations discussed throughout this essay.
The day chosen for this study is August 11th which has a day-length of 15 hours (Birmingham, UK. Table 2). This day is considered to be typical of a range of days in the campaigning season and for different latitudes within northern Europe. The campaigning season is roughly defined as the grass-growing season: that is April to October for the mid-UK.
Although this essay is specifically targeted at understanding the variables that controlled a legionary day in Britain, it will become clear that it also describes, and places significant limits on, the activity of legionaries operating in much warmer climates, e.g. the Mediterranean, north Africa and the Middle-East. Additionally, the day-length of 15 hours matches the maximum for Rome in late June which, of course, means that the times and possible duration of marches in this essay also apply to mid-Mediterranean latitudes.
|
Twilight Sunrise |
Sunrise |
Sunset |
Twilight Sunset |
Day length |
Twilight to twilight |
|
05:04:00 |
05:50 |
20:34 |
21:09 |
14:59 |
17 hours (approx.) |
Table 2: Significant hours and time lengths for August 11th, Birmingham, UK.
Table 3 shows the schedule of typical tasks performed by a legionary during August 11th. For this example the marching army is one legion in number (5120 soldiers) and is using a Roman road to move from one temporary marching camp to another.
Many authors when creating a description of a Roman marching column place scouts, pioneers and cavalry units at the front as a reconnaissance unit, followed by the vanguard of auxiliary troops, some legionaries and the camp surveyors. The vanguard might be followed by the command group: the army commander, his senior officers, guard and support staff. Then comes the main body of the army composed of the legionaries, auxiliaries and much of their baggage. Finally there may be a rump force, or rear guard, of some baggage, auxiliaries and a cavalry escort. What is apparent from this description is that the reconnaissance, vanguard, command group and the rear guard are by their operational nature small, fluid units, whereas the main body, the soldiers and their baggage, is much larger and essentially fixed in size and extent once the march commences. For example, in extremis the outliers (vanguard etc.) might retreat, or otherwise seek shelter, within or behind the main body the main fighting units. Additionally, it was the main body that carried out most of the physical work related to the building of a new camp; and it was its march and work-rate that determined when tasks were completed and whether or not all tasks could be safely completed before the end of the evening twilight. Further more, the time gaps that would have existed between these smaller units and the main body are not known. Would the reconnaissance unit have been separated by 10, 15, 30 or more minutes from the vanguard, and would that body, which contained the camp surveyors, be similarly separated from the main body? Clearly these gaps would have depended on circumstance: for example, the prospect of contact with an enemy shortening them. Therefore, a most likely time gap of 10 - 15 minutes between the camp surveyors beginning laying out the new camp and the arrival of the first units of the main body that would then start to dig the camp, is not very important in the context of the 24 hour period under discussion, or the two, three or four hours it might take to build the camp (see Appendix: The building of temporary marching camps).
Therefore, in this study the composition of the army, its lay-out, its length and timed values are based on the main body of the army, that is the soldiers and their baggage.
The activities throughout the day shown in Table 3 are based on a common understanding of the task at hand, namely, prepare for an approximately 7-9 hour march, build a marching camp and then eat, repair equipment and sleep in preparation for repeating the process the next day. Some specifics are based on John Peddie's, 'The Roman War Machine' (Peddie, 1994). The finer details of camp life have not been included in this table, but allowance has been made within the timings for such activities.
As an aside, the late John Peddie, OBE, MC, was a British Army infantry officer, served in India during WWII, had experience of marching columns that used mules, and very importantly, had access to British Army statistics on, for example, the time it takes for an infantry man to dig a ditch. In short, much of this essay and the many calculations that underlie it, owe their provenance to John Peddie's work.
The day shown in Table 3 starts at morning twilight with an hour for personal grooming and preparation for the day ahead. At sunrise breakfast is taken before the camp is vacated by the first units at 7:00 h. At a velocity of 1.2741 m/s (4.59 kph or 2.85 mph – the default on-road velocity figure, Whip, 1998) the legionaries cover 29 km (a figure commonly assumed to be the legionary day-rate of march) in 7:22 h, the first unit arriving at the night-stop at 15:22 h. A rest-period of 10 minutes per hour is included in the hours spent marching and at an energy expenditure (EE) of 132 kilocalories per hour (henceforth shown as kcal/h); this value probably errs on the low side, but the author has failed to find figures for decreasing EE as the human rests after work.
Generosity is granted
this example legionary in that he has eight hours of sleep
uninterrupted by guard duty. For larger armies this arrangement may
have been the case because, as we shall see, there were sufficient
soldiers available to allow a separation of duties: in this case,
those who dug the ditch and rampart may have been excused guard duty.
|
Time (24 h clock) |
Events |
Legionary activities |
Energy Expenditure kcal/h |
Horse and mule grazing |
Notes |
|
5 |
Morning twilight |
Personal grooming |
176 |
Grazing |
|
|
6 |
Sunrise |
Breakfast. Break camp. |
264 |
|
|
|
7 |
|
Start march |
400.60 |
|
First unit leaves camp |
|
8 |
|
March |
400.60 |
|
Last unit leaves camp 1:23 h after first |
|
9 |
|
March |
400.60 |
|
|
|
10 |
|
March |
400.60 |
|
|
|
11 |
|
Midday meal and break |
132 |
Grazing |
|
|
12 |
|
March |
419.68 |
|
|
|
13 |
|
March |
419.68 |
|
|
|
14 |
|
March |
579.16 |
|
|
|
15:22 |
|
Arrive at new camp. Dig ditch and build rampart |
530 |
Grazing |
Ditch and rampart takes 2-4 hours. |
|
16 |
|
Erect tent |
264 |
Grazing |
Last unit arrives at 16:46 h. |
|
17 |
|
Personal grooming |
176 |
Grazing |
Camp complete. |
|
18 |
|
Evening meal |
132 |
Grazing |
|
|
19 |
|
Rest and repairs |
88 |
Grazing |
|
|
20 |
Sunset |
Rest and repairs |
88 |
Grazing |
|
|
21 |
Evening twilight |
Sleep |
79.25 |
Place animals in camp |
|
|
22 |
|
Sleep |
79.25 |
|
|
|
23 |
|
Sleep |
79.25 |
|
|
|
24 |
Midnight |
Sleep |
79.25 |
|
|
|
1 |
|
Sleep |
79.25 |
|
|
|
2 |
|
Sleep |
79.25 |
|
|
|
3 |
|
Sleep |
79.25 |
|
|
|
4 |
|
Sleep |
79.25 |
|
|
|
|
Total Kilocalories |
|
5504.92 |
|
|
Table 3: An example schedule for one legion marching along a Roman road (modern dirt road). The kcal/h energy expenditure figures are for an 80 kg legionary, marching at 1.2741 m/s (4.59 kph or 2.85 mph), carrying a 40 kg load over a level, Roman road. Note 1: the term 'dirt road' is from US Army marching studies. A dirt road typically matches the gravel walking surface of the majority of Roman roads. Note 2: the kcal/h value for the last marching hour at 14:00 hours is increased to 579.16 kcal/h to account for the 7:22 hours taken to march the full 29 km, i.e. 0:22 hours has been added to the calculation at 14:00 hours. Note 3: most of the kcal/h figures, except for marching and digging, were taken from http://www.caloriesperhour.com/index_burn.php .
This typical schedule is matched by a fully-matured legionary who, the ancient writers suggest, was 176 cm tall (5 ft 10 inches) and weighed approximately 80 kg (176 pounds or 12st 8.4 lb). These physical attributes are factors in the calculation of energy expenditure, for example, an 80 kg man will expend more energy completing a task than one weighing 60 kg - even sleeping.
Researchers have noticed, but not yet measured, that men marching for extended hours show an increase in energy expenditure per hour over the standard measured rate, i.e. to march further, for longer, costs more each hour. It is thought this might be due to a combination of tired muscles allowing the body to become increasingly unbalanced which requires more energy to correct, and increased energy expended in thermo-regulation as the hours progress. Estimates of this increase vary between 10 and 16% for endurance marching. In Table 3 the effect is replicated by increasing the EE in the morning my 5% per hour and 10% per hour in the afternoon, i.e. an increase of less than 10% for the whole march, and therefore equal or lower than researchers' estimates.
Accurate measurements of the total weight of all items placed on the body of the naked legionary are fraught with uncertainties of many kinds, and much effort by many academics and others, has been expended trying to produce plausible weights (Roth, 1998). The generally accepted weights for all items, excluding rations, is approximately 30 kg (some authors produce lower figures, others higher, but 30 kg is a reasonable compromise). Assuming that the daily ration weight was 1.1 kg, then approximately 10 days of rations would have weighed 11 kg which brings the total weight carried on the naked body to 41 kg. Rounding down this figure to 40 kg produces a plausible figure that the legionary was trained and required to carry; the total, marching weight of the legionary in Table 3 is therefore 80 kg plus 40 kg, 120 kg.
Interestingly, the soldier in the modern British Army is required to carry a 'Marching Order' load of 40.2 kg; this consists of all clothing, helmet, weapons, ammunition, a digging tool, food for at least 24 hours, rucksack (Bergen) and a sleeping bag. This similar figure supports that assigned to the Roman legionary.
In the column 'Notes' (Table 3), the last man leaves the marching camp 1:23 hours after the first: this is the time it takes for the whole army (soldiers, mules and carts) to exit the camp, or for the marching column to pass by a fixed point. The time taken to exit the camp in all calculations in this essay derives from the simplest exiting strategy, namely, a set march velocity for different types (soldiers, carts and mules) and the number of gates in operation. For the case in Table 3, where an army of 1 legion exits and joins a single road, the time taken for any movement of the army is governed by the speed of march and access to the road: the road access point is essentially acting as a gate - the bottleneck. However, beyond this simplest case, more complex exiting strategies could have been deployed by the Romans, some that would delight a modern-day Regimental Sergeant Major, but the overall effect would not greatly diminish the bottle-neck and are not considered in this essay. Also, no additional time is allocated to forming-up the marching units either within the camp or externally, it being assumed that the units would compensate for such short delays by a quicker, initial pace.
At 15:22 hours the first legionaries arrive at the new camp and some start to dig the ditch and build the rampart that will surround the camp. Depending on the depth of the ditch this might take 2, 3, possibly 4, or more hours (see Appendix). However, this study presumes that a legionary might only participate for 60 minutes, but probably less, before being relieved: to do otherwise would dramatically increase the legionary's daily energy expenditure beyond a point that is sustainable if repeated day-after-day. Also, the 530 kcal/h figure for digging in Table 3 is the lowest of those found in the literature: other examples are 630 and 748 kcal/h.
This study replicates the numbers of servants, mules and horses per legion as used in earlier essays by the author (see www.bandaarcgeophysics.co.uk/arch_intro.html ). Each contubernium (eight soldiers sharing a tent)was supported by at least two servants, and the same number of mules used as baggage transport. The baggage transport may have been a mix of pack-mules and mule-carts (2 mules pulling a 2 wheeled cart – after Peddie, 1994). To be clear, these mules form the troop-baggage-train, that which marched with the legion, and not the army-baggage-train which would be located in the rear supply-area and contained reserve food-stuffs, equipment and siege machines.
Peddie estimated the troop-baggage-train contained 1,375 mules per legion, while the author's figure used in this essay is 1,280; the difference is not significant given the inevitable variability in the historical figures.
Feeding horses and mules while campaigning was a difficult and crucial business: if the mules become incapable of transporting the army's goods then it could not conduct a campaign of movement. It is for this reason that Roman armies generally did not campaign until the start of the grass-growing season. An animal – horse or mule - that is 14 to 15 hands tall and weighs approximately 400-450 kg, consumes roughly 10 kg of dry fodder (hay or cut-grass) a day. For a single legion that amounted to 12.8 metric tonnes for the mules alone. Some of this could have been replaced by grain transported by the mules or by allowing them to graze. Some dry fodder could have been collected by the legionaries, each of which carried a scythe. Parties of soldiers would collect the fodder from fields and transport it into the camp for the tethered animals. Using this method the benefits are those of time saved, security and management: time saved because men could collect the required fodder from the field faster than a horse or mule could eat the equivalent; security because groups of men collecting hay outside of the marching camp were easier to protect than a barren of mules; and management, in that the mules and horses could be securely tethered within the camp and fed there, even after night-fall.
Nevertheless, grazing was important. Peddie stated that the 10 kg basic requirement could be gained from at least five hours of grazing. In Table 3 are shown 8 hours of possible grazing time, suggesting that if fodder and grain were not available the mules and horses could still find sufficient to sustain them.
It is probable that all three methods of animal feeding – grain, fodder and grazing – took place within a single day in ratios that were dependent on the security of the camp and other factors such as the season. It is interesting to note that, according to the preceding calculations, the animals of the troop-baggage-train were not dependent on supply from the army-baggage-train; not so the soldiers, whose carried supply of grain and other comestibles could have been supplemented by foraging etc., but would eventually need replenishing by visiting a grain store at a fort or by the army-baggage-train.
Nearly all of the numbers produced in this essay arise from the computations of various variables held in a spreadsheet; these are displayed in Table 4.
The numbers for each type
of unit (soldiers, either legionary or auxiliary, pack-mules and
mule-carts) have been used to calculate the length of units as they
marched which, when appropriately combined with the march velocity,
gives various time and duration figures, e.g. the total length of an
army column, the time it then took to pass a single point, the time
it took to exit a camp and the arrival times at the new camp.
|
|
Default Variable |
Variations and Notes |
|
Number of legionaries in legion |
5120 |
Typically assumed a standard for 1stC AD. |
|
Legionary body weight |
80 kg |
Well built male, 5ft 10in tall, 26-32 years old. |
|
Legionary carried weight |
40 kg |
All clothing, armour, arms, personal equipment and food-stuffs. |
|
Total legionary weight |
120 kg |
Combination of body and carried weights. |
|
Factor for marching terrain |
1.1 |
1.0 black top road; 1.1 dirt road; 1.2 light brush; 1.5 heavy brush; 1.8 swampy bog; 2.1 loose sand; 2.5 soft snow 15 cm; 3.3 soft snow 25 cm |
|
Marching velocity (on-road) |
1.2741 metres/sec (2.85 mph or 4.59 kph) |
Pack-mules and mule-carts move at the same rate. |
|
March start time |
07:00AM |
|
|
Time allowed for midday meal |
1 hour |
|
|
Time for rest-period per hour |
10 minutes |
|
|
Terrain slope |
0 |
The slope of the terrain over the march route is kept at 0.0 in most calculations. |
|
Marching velocity (off-road) |
0.6706 metres/sec (1.5 mph or 2.414 kph) |
US army 2.414 kph. |
|
Legionary rank space |
2 metres |
Distance between ranks |
|
Legionary file number |
6 |
Six is typically assumed to be a standard |
|
Distance between centuries |
4 metres |
Approximation by author – enough room for the centurian's horse. |
|
Number of carts per legion |
158 |
Scaled from Peddie, 1994. |
|
Number of mules per cart |
2 |
From Peddie, 1994. |
|
Mule-cart length |
9.14 metres |
Includes mule length. From Peddie, 1994. |
|
Mule-cart file number |
2 |
From Peddie, 1994. |
|
Number of pack-mules per legion |
964 |
Scaled from Peddie, 1994. |
|
Distance between carts |
2 metres |
An approximation by author |
|
Number of pack-mules per legion |
964 |
Scaled from Peddie 1994. |
|
Pack-mule length |
4.572 metres |
From Peddie 1994. |
|
Pack-mule file number |
2 |
From Peddie 1994. |
|
Distance between pack-mules |
1 metre |
An approximation by author |
|
Animal dry fodder per day |
10 kg |
Animal weight 400-450 kg |
Table 4: variables used in various calculations
throughout this essay.
As an example of some of the calculations Table 5 shows various values for legions marching along a road at 1.2741 m/s (2.85 mph, 4.59 kph) with a 10 minute rest each hour. The table extends to 16 legions, 81,920 soldiers, because the data are instructive of the difficulties faced by the Romans in managing extremely large armies, for example at the battle of Cannae. In Britain the largest, known group of temporary marching camps suggest the the number of legions occupying was 9+, amounting to 46,080 soldiers, i.e. legionaries and auxiliaries. These camps form the Group 65-70 hectares (see “Roman Marching Camps in Britain: GIS, statistical analysis and hydrological examination of known marching camps, resulting in the prediction of possible camp sites." for a detailed discussion on British marching camps).
The final column, 'Time for the army column to pass', shows that it would have taken 1:23 h for a single legion to march past a point, 9 legions 12:34 h and, finally, 16 legions 22:21 h; clearly for a Roman army that moves as a single entity these latter figures were not tenable. They arise because of the basic requirement, of this set of calculations, for all units to march in a single column along the road. This table hints at two basic facts which will be examined in detail later: 1) Roman armies of 1 and 2 legions in size could have marched in single column along a road; 2) armies greater than 2, possibly 3, legions marched in multiple columns whether on- or off-road.
|
Legions # |
Soldiers # |
Soldier column length (metres) |
Carts # |
Cart column length (metres) |
Pack-mules # |
Pack-mule column length (metres) |
Total army column length (metres) |
Time for army column to pass (h) |

Table 5: Some calculations for legions marching in single column along a Roman road. March velocity 1.2741 m/s (2.85 mph) using the example legionary from Table 3. Rest-period of 10 minutes/hr. Calculations in part derived from Table 4. The term 'soldiers' does not differentiate between legionaries and auxiliaries: both, probably, marched in a similar manner.
The length values for the various types of units - soldiers, carts and pack-mules - have a direct and obvious bearing on the time it would take an army to exit a marching camp or pass a single point. In the case of armies marching in single column along a road the time for the army column to a pass a single point (Table 5, last column) is equal to the time to exit the camp: this is because the benefit of exiting from multiple camp-gates is negated by the need for units to wait for the preceding units to join and clear the road. To re-emphasize an earlier statement, the point at which units join the road is the bottle-neck, not the camp exits as one might expect. Not so for armies marching off-road, or large armies that could not march in single column and arrive at the new camp in a reasonable period of time, and hence, would use multiple camp exits and columns. (Note: there is an exception where a road exists between the marching camps thereby allowing the units to consecutively join the road having marched part of the distance off-road: in essence this causes the bottle-neck for joining the road to continually move towards the next camp. This special case is examined later.)
Table 6 shows the exit calculations for armies using multiple gates and then proceeding to march in multiple columns, i.e. the units exiting do not have to wait for preceding units to join and clear a road or single column (compare to Table 5, 'Time for army column to pass').
|
Legions # |
Soldiers exit time (mins) |
Carts exit (mins) |
Pack-mules exit time (mins) |
Total exit time (mins) |
Total time to exit (h) |

Table 6: Example times to exit a marching camp for armies leaving via multiple exits and joining multiple columns. Velocity 1.2741 m/s (2.85 mph). Each type of unit - soldiers, carts and pack-mules – respectively, leaves via 2, 2 and 3 gates simultaneously.
The use of multiple exit gates, allowing the issue of units into multiple, parallel columns, greatly diminished the time taken to exit the camp and begin the march; Table 6 demonstrates one reason why Roman armies often built multiple gates in each side of their marching camps.
To further this discussion of the methods employed in this essay the results of Table 6 can be coupled to an example of one variation of multi-column marching, namely, Table 7 shows the same parameters as those in Table 5 for single-column marching but for armies with 2 columns of carts, 2 columns of pack-mules, and this block of four columns enclosed on its side by columns of soldiers, i.e. the troop-baggage-train is flanked by parallel-marching units of soldiers.
|
Legions # |
Soldiers # |
Soldier column length (metres) |
Carts # |
Cart column length (metres) |
Pack-mules # |
Pack-mule column length (metres) |
Total army column length (metres) |
Time for army column to pass (h) |

Table 7: Calculations for legions marching in multiple columns. March velocity 1.2741 m/s (2.85 mph) using the example legionary from Table 3. rest-period of 10 minutes/hr. Calculations in part derived from Table 4. See the text for further details.
For this parallel, multi-column marching method (Table 7), the unit with the longest length, i.e. the pack-mules at 1324.85 metres for a single legion, determines the time it takes the army to pass a single point, that is 00:17 h; in contrast, a single legion in single column (Table 5) takes 1:23 h. Immediately one can appreciate the benefits of multi-column marching, especially where very large armies are concerned – compare the 16 legion figures in Tables 5 and 7, 22:21 and 04:41 h, respectively. Of course, this comparison exercise is moot because multiple columns did not have access to multiple, parallel roads, and therefore, would not have had a march velocity of 1.2741 m/s. Nevertheless, the example demonstrates the obvious advantages of multi-column marching.
The discussion in this section demonstrates the variety, possibilities and limits that differing strategies could have given the Roman army when moving from camp to camp. The benefits of using a spreadsheet to keep computations between different formations comparable is obvious; the drawback is the rather large number of values and variations in formations one would like to discuss but are impractical in an essay.
Energy expenditure and on-road marching velocity of Roman legionaries.
The 20th and 21st century military have spent time and money on trying to understand the energy expenditure (EE) of soldiers marching under various loads. In part this effort has resulted from a number of embarrassing episodes where soldiers struggled in their allotted tasks due to not being march-fit or having loads that grossly exceeded their carrying capacity; these types of events are still occurring.
The primary work to derive an empirical formula describing marching was conducted by K.B. Pandolf and co-authors of the US Army Research Institute of Environmental Medicine in 1976-7 (Pandolf, 1976); this work is still in use today and can be used to gain an understanding of how a legionary operated.
Pandolf's EE equation is:
Mw = 1.5 • W + 2.0 • (W + L) • (L / W)2 + T • (W + L) • (1.5 • V2 + 0.35 • V • G)
where:
Mw = metabolic cost of walking (watts); W = body weight (kg); L = load weight (kg); T = terrain factor; V = velocity or walk rate ( m/s); G = slope or grade (%)
Terrain factors: 1.0 = black topped road; 1.1 = dirt road; 1.2 = light brush; 1.5 = heavy brush; 1.8 = swampy bog; 2.1 = loose sand; 2.5 = soft snow 15 cm; 3.3 = soft snow 25 cm; 4.1 = soft snow 35 cm.
In this essay this equation has been used to calculate all the EE figures and graphs; note that the dirt road value of 1.1 is used to mimic a Roman road and that the slope value is always set to 0.0, i.e. flat unless stated otherwise. The use of the terms 'light brush' and 'heavy brush' are specific to North America and do not translate clearly to the vegetation types and agricultural practices of Britain or northern Europe in the 1st century AD. Hence, for legionaries marching off-road a terrain factor of 1.35 is used in this essay – a reasonable mid-point between light and heavy brush. Finally the metabolic cost of walking in watts is converted to kilocalories per hour (kcal/h) by the multiplier 0.859845227858985.
The relationships between work (marching, digging etc.), heat production, energy expenditure and de-hydration (sweating) and the replacement of fluids is explained (in part) by the following: 1 kcal is the energy required to raise the temperature of 1 kg of water by 1 degree Celsius; 540 kcal evaporates 1 litre of water.
For our example legionary weighing 80 kg, carrying a load of 40 kg, at a march-rate of 1.2741metres/sec (2.85 mph) over a dirt road, the calculated EE is 501.42 watts or 381.53 kcal/h. Over a 7:22 hour march (which includes a 10 minute break every hour, but not hour-long meals) he would have covered 29 km at an EE of 2814 kcal. However, this value does not include a factor for fatigue, which at +10% of that calculated, increases the EE to 3095.68 kcal.
Using the same variables, Table 8 shows the calculations of EE for legionaries weighing 60 to 90 kg.

Table 8: The energy expenditure (EE) of marching legionaries of differing body weight. Load 40 kg, march velocity 1.2741 m/s and marching 7:22 hours over a Roman road to cover 29 km. The 'EE kcal/h' figures include a 10 minute rest at 2.2 kcal/min.; the 'kcal per march' figures are simply the EE kcal/h figure multiplied by the number of marching hours (7:22); the 'kcal per march +fatigue' values are simply 10% increases of values in the previous column.
The EE difference between the 60 kg and 90 kg legionaries (Table 8) is significant and illustrates how the movement of differing masses through the Earth's gravity field has a variable energy cost. Simply, whatever the 60 kg legionary accomplished, the 90 kg man used and required more energy and water for the same accomplishment. The additional requirement of the heavier man, i.e. more food and water, can be significant and if lacking can have detrimental effects on the health and military efficiency of the soldier.
As an interesting aside relevant to this issue, and indicative of how critical sufficient food is to hard-working soldiers, the US Army while operating in Afghanistan discovered that they were inadvertently starving their special force soldiers: they reported a loss of weight, energy and capability, although the reason was not understood, until it was realised that their EE/day was not being replenished by the standard food portions dispensed by the army canteens. The solution was to give the special force soldiers extra rations to match their EE. Hence, one can understand why Caesar, when writing about his campaigns in Gaul, frequently discusses his supply-chain.
In contrast to the US Army soldier, the legionary was expected to be partially independent of the supply train. As already discussed legionaries (and presumably auxiliaries) carried hand-scythes for gathering food and fodder. Generalised foraging was a common activity of the legionary force and might have been organised on the 8 man team (contubernium). In that manner, the heaviest man, he who required the greatest amount of food, might have been supported by his lighter colleagues; however, this is speculation, there being no mention of this in the ancient sources. Of course, the heavier, stronger man would reward his colleagues at the most critical of times.
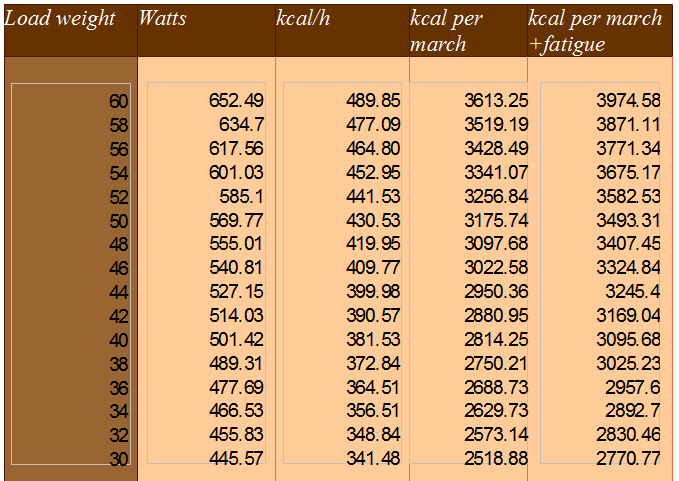
Table 9: The energy expenditure (EE) of the example legionary but with varying load weights. Other parameters the same as Table 8.
In Table 9 the EE of the example legionary with varying load weights is shown, while Table 10 contains the EE for different on-road velocities. The information combined in Tables 8, 9 and 10 demonstrates the considerable differences in EE for differing body weights, load weights and march velocities. It is clear that for any length of march the heavier men would have had to work much harder than the example legionary at 80 kg body weight. Conversely, the lightest men at 60 kg, and an adverse body/load (40 kg) ratio of 3/2, might have struggled to manage their loads, but their EE would have been low.
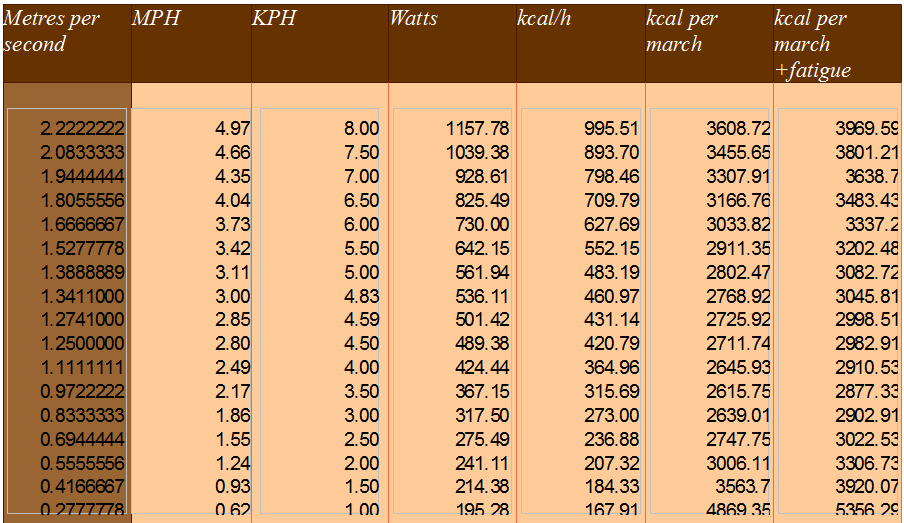
Table 10: Energy Expenditure of the example legionary (body weight 80 kg, load 40 kg) for varying road march velocities. The example legionary has a selected march velocity of 1.2741 m/s; US Army and Marines is 1.111 m/s; British Army is 1.3411 m/s. No rest-periods have been factored into this table.
Table
11 shows the EE figures for a legionary weighing 90 kg, load weights
at 50 and 55 kg, and march velocities of 1.2741 m/s (2.85 mph, US
Army) and 1.3411 m/s (3.0 mph, British Army). As we shall discuss in
a later section, any EE above 500 watts can be considered as
potentially dangerous with any activity above this figure requiring
careful management of rest-periods, water intake and food to avoid
heat-stress. The very high watt levels suggest that weights of 50 or
more kilogrammes were not carried and that a maximum weight limit of
40 kg for all soldiers was likely. Coincidentally, the figures in
Tables 9 and 11 are an explanation of why modern soldiers, frequently
overloaded with weights exceeding 50 and 55 kg, perform poorly and
fail to complete tasks – a feature frequently commented upon by
modern army medics and others.
|
Velocity (metres/sec) |
Body weight (kg) |
Load weight (kg) |
Watts |
kcal/h |
kcal per march |
kcal per march +fatigue |
|
1.3411 |
90 |
50 |
636.88 |
478.66 |
3354.33 |
3689.76 |
|
1.3411 |
90 |
55 |
673.61 |
504.99 |
3538.84 |
3892.72 |
|
1.2741 |
90 |
50 |
596.41 |
449.64 |
3316.65 |
3648.31 |
|
1.2741 |
90 |
55 |
631.69 |
474.93 |
3503.22 |
3853.54 |
Table 11: The energy expenditure for a legionary weighing 90 kg, marching at either 1.3411 or 1.2741 metres/sec. and carrying loads of 50 or 55 kg. A rest-period of 10 minutes is factored into the kcal values. Compare with Tables 8, 9 and 10.
For example, although the US military authorities stipulate that: “The fighting load should not exceed 48 pounds (~21 kg), and the approach march load (that includes the fighting load) should be less than 72 pounds (~32 kg)….” (source: Foot Marches. FM 21-18, US Depart. Of Army. 1990), nevertheless, US soldiers regularly carry loads far in excess of 32 kg. One recent study of an US regiment found that on average the approach march-load was 46 kg, and the emergency approach march-load 60 kg, with extremes up to 68 kg (source: The Modern Warrior’s Combat Load - Dismounted Operations in Afghanistan. Task Force Devil, Coalition Task Force 82, Coalition Joint Task Force 180. 2003). It is known that carrying these excessive weights is not sustainable as a normal operational routine, and the US army recognises the urgent need to decrease the load carried by its soldiers. The critical point to be taken from these modern observations is that the physiology of the Roman soldier would not have been very different to his modern equivalent: hence, if the modern soldier cannot cope with weights much above 40 kg, then neither could the Roman soldier.
The Pandolf equation for the example legionary can be applied to a grid of grade/slope values of all known Roman roads in Britain (Figure 1). The result is a range of EE from 501 to 542 watts (431 to 466.35 kcal/h (neither rest nor fatigue included)). This range of values can be examined with regard to induced heat stress in the legionary (see Figure 2).
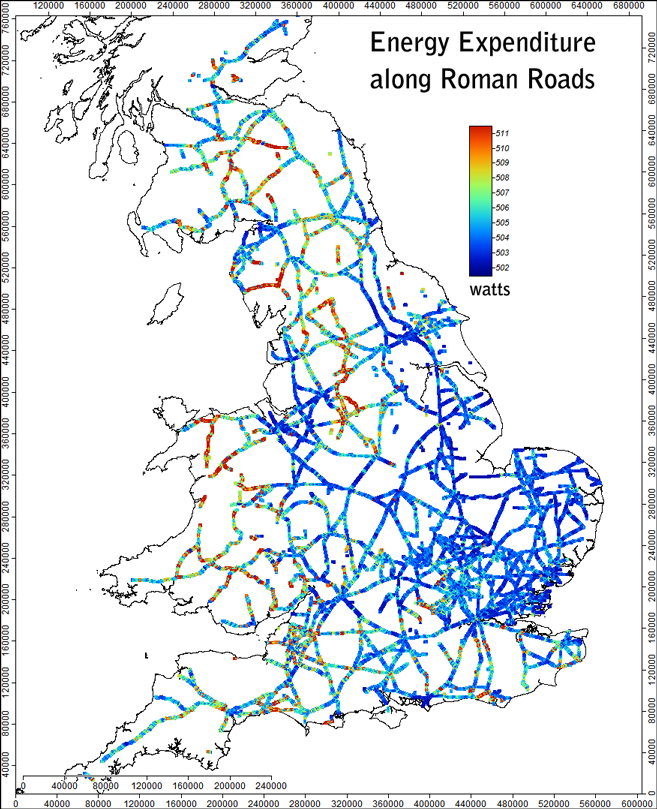
Figure 1: Energy expenditure (watts) for Roman soldiers marching along Roman roads. Total range is 501 to 542 watts for the example legionary (body weight 80 kg, load weight 40 kg, march velocity 1.2741 m/s). The Pandolf equation (Pandolf, 1976) has been applied to slope% values derived from SRTM data along the Roman roads. The road surface value is set at 1.1, a dirt road; if the value had been 1.0, a black-top road, then the lowest value drops to 472 watts.
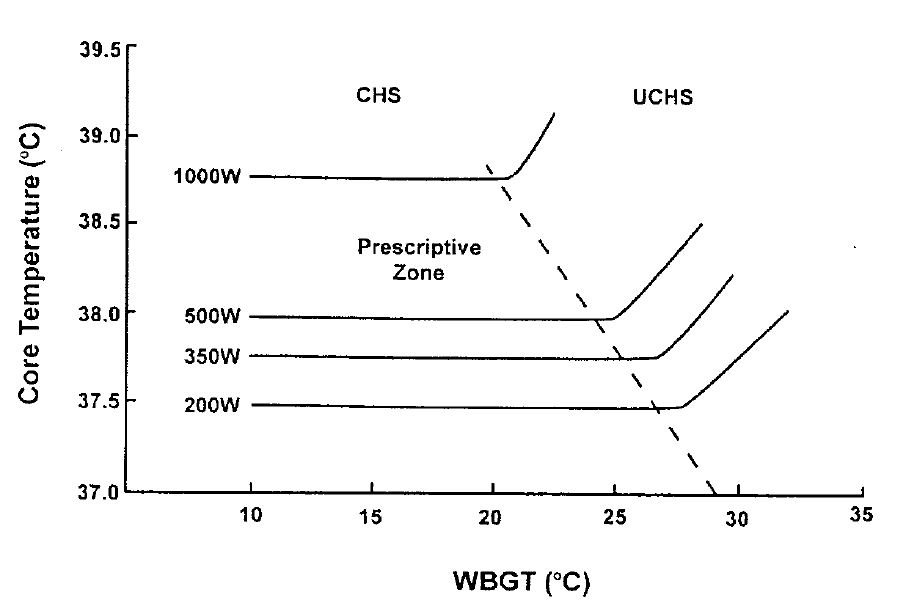
Figure 2: Core temperature (steady state) responses during physical work for Compensated and Uncompensated heat stress. Source: Heat Stress control and Heat Causality Management, Technical bulletin medical 507, US Air Force 48-152(1), 2003. WBGT is wet bulb global temperature. Compensated Heat Stress (CHS); Uncompensated Heat Stress (UCHS). See text for description.
A
brief description of Figure 2 is necessary. It shows steady-state
core temperature responses at several metabolic rates (i.e. EE at
200, 350, 500 and 1000 watts). As we have seen, the Pandolf equation
demonstrates that metabolic rate during marching is dependent on
speed, terrain (slope and surface) and load carried. Compensated
heat stress (CHS in Figure 2) exists when heat loss occurs at a rate
in balance with heat production, i.e. the stress is manageable and
not debilitating. Uncompensated heat stress (UCHS in Figure 2) exists
when a steady-state core temperature cannot be attained and rises
until exhaustion of the subject. The dashed line in Figure 2 is the
boundary between CHS and UCHS.
With reference to Figure 2, in a British Summer the day-time temperatures typically range from 20-25 °C but with a high humidity; average, August, relative humidity for Birmingham is 80%. The example legionary would have expended 501-542 watts when marching along British roads (Figure 1); these figures show that the legionary was constantly working at high wattage, close to the dashed boundary between CHS and UCHS. For the hottest of days (or further south, or east, in the Roman Empire) the example legionary would operate closer to the dashed boundary: care would have been needed to ensure he did not become heat exhausted. For a legionary weighing 90 kg the situation was even more critical; the work rate was high enough (Table 8, 534 watts) to induce exhaustion unless this individual was well acclimatised, frequently rested, fed and hydrated. Furthermore, and to re-emphasise the point made earlier, the very high watt values in Table 11, 596-673, (legionary weighing 90 kg, velocity 1.3411 or 1.2741 metres/sec., loads of 50 or 55 kg), all of which are in the 'prescriptive zone', strongly suggest that these high load weights were not normally carried.
Many other combinations of velocity, load, rest-periods etc. could be discussed to demonstrate how carefully balanced the CHS side of the dashed boundary (Figure 2) the marching legionary force was in warm weather, but to do so would become onerous for the reader. Suffice to say that the Roman army was apparently as well practised and trained in marching techniques, as they were in many other military aspects.
The document 'Heat Stress control and Heat Causality Management, Technical Bulletin Medical 507, US Air Force 48-152(1), 2003' (the source for Figure 2) contains a table, 'Table 3-1', for fluid replacement and work/rest guidelines for US Army soldiers, heat-acclimatised, wearing battle-dress uniform and operating in differing temperature regimes. Table 3-1 has been metrically converted and adapted as Table 12.
|
|
Moderate Work (425 Watts) |
Hard Work (600 watts) |
||
|
WBGT (wet bulb global temp °C) |
Work/rest (minutes) |
Water Intake (ltr/hour) |
Work/Rest (minutes) |
Water Intake (ltr/hour) |
|
25.5 - 27.72 |
No limit |
0.71 |
40/20 |
0.71 |
|
27.78 – 29.39 |
50/10 |
0.71 |
30/30 |
0.95 |
|
29.44 – 31.056 |
40/20 |
0.71 |
30/30 |
0.95 |
|
31.11 – 32.17 |
30/30 |
0.71 |
20/40 |
0.95 |
|
> 32.22 |
20/40 |
0.95 |
10/50 |
0.95 |
|
|
|
|
|
|
Table 12: Re-hydration and work/rest rates for warm weather conditions. Source: Table 3-1 of Heat Stress control and Heat Causality Management, Technical Bulletin Medical 507, US Air Force 48-152(1), 2003. WBGT is wet bulb global temperature. See text for description.
The calculations in Table 12 are limited to a 4 hour duration, and probably underestimate the figures for the example legionary in Table 3 who required 7:22 hours to cover a 29 km march. Additionally, the example legionary was expending 501–542 watts while marching across Britain, figures that fall between the Moderate and Hard work-rates of Table 12, hence, the closer figures of the Hard work-rate examples will be used in the following discussion.
Furthermore, Bulletin 507 (Table 12) states that, for individuals wearing body armour in humid conditions, that the examined temperature range should be increased by 5 degrees. Unfortunately, the Bulletin does not specify a high, relative humidity figure (the specification is based on “observed dripping sweat”), however, it seems reasonable to assume that the typical, British Summer, relative humidity of 80% (+/-5%) would qualify. Most readers will be aware from television images of the form, and close fitting nature, of modern body armour, and can readily appreciate how restrictive these garments are to the evaporative properties of the body. In general, the lower the evaporation rate, the lower the cooling which, in turn, causes the body to sweat more, hence use more energy, and then requires even more hydration to avoid a critical temperature rise into the UCHS previously discussed (of course, the assumption is that other protective measures beyond increased hydration, such as a decrease in march velocity, or an increase in the rest-period per hour, have not been applied). But what of the legionary?
His large, curved shield was probably encased in a harnessed leather bag that allowed the shield to be carried on his back, with his pack (sarcina) of belongings and rations attached to a long pole, with a cross piece at one end (furca) where the pack was attached, allowing that to rest on the top of the shield. This arrangement is similar in form and function to the modern infantryman's back-pack. Of course, the legionary clothing and armour would have been different, but the consequences would have been similar. Next to the skin of his torso and arms he probably wore a light linen shirt, and on top of that a woollen tunic, while his exterior would have been of metal: a ring-mail shirt (lorica hamata), or a suite made of strips and sheets of iron (lorica segmentata). Between the woollen tunic and metal-outer may have been a thick jerkin (subarmalis), thought to have been made of felt, leather or thickened linen which was designed to help the metal armour fit the body, stop chafing, and to absorb and distribute the energy of blows to the metal-outer. Necessarily this garment was tight fitting and, due to the weight of the metal armour, compressed onto the torso. Even if the jerkin was not being worn the weight, and non-porous nature of the metal-outer, would have limited evaporation. It seems likely, therefore, that the legionary was as enclosed while marching as the modern infantryman wearing body armour, and would suffer similar limitations to effective evaporation of sweat. This has two direct effects on the use of Table 12.
First, for the example legionary this ruling means that all actual WBGTs in Table 12 have to be increased by 5 °C, pushing the Work/Rest and Water Intake values to be considered up two temperature rows. Second, the first WBGT range, 25.5 - 27.2 °C, is that which should be applied to a legionary marching at 20 °C, i.e. a temperature commonly reached during many days in Spring, early and late Summer, and early Autumn in Britain. Of course, for the second case, the number of hours in a day when the WBGT reached 20 °C would have been fewer than in the height of Summer.
Yet another aside: the pole to which the legionary attached his belongings, and then perched on top of his back-carried shield, may have been more energy efficient than the modern-day rucksack. The pole would pass over the shoulder which would act as a pivot allowing one of the legionary's arms to act as a counterbalance to the weight of the belongings. Consequently the centre of gravity of the belongings would shift towards the shoulder, or mid-plane, of the legionary body, a position that would place more of the weight of the load over the legionary hips. This would have a number of effects. First, there would be a small energy saving for each step which, when multiplied by the thousands taken each day, would have been significant. Second, the movement of the centre of gravity would have improved the legionary's posture and breathing: most modern soldiers bend forward at the hips to bring their centre of gravity over their hips, creating a restriction in their breathing, albeit small, and all sorts of physiological stress to the spine, neck and associated muscles, tendons and ligaments. Furthermore, with the legionary load pivoted on one shoulder, albeit with most of it distributed across both shoulders and back by being rested on the top of the shield, would have been counter-balanced by the weight of the javelins (pila) carried on the other. Consequently, compared to the modern soldier, the legionary might have walked more upright for the same carried load, avoided some of the physical complaints, and breathed more easily, thereby decreasing his EE. (Note: this hypothesis is not fact until an empirical study is conducted.)
A further ruling in Bulletin 507 states that the water intake values are dependent on the needs of the individual, exposure to full sun or full shade, and are variable by +/- 0.47 ltr/h. Thus, some soldiers might require 1.18 ltr/h when doing hard work in a WBGT of approx. 25-27.7 °C; the example legionary might have required 8.71 litres of water to complete his 7:22 hour march. If the applicable WBGT range rises above 27.78 °C then the water requirement might rise to 1.42 ltr/h or 10.47 litres for the march. But, it is important to remember the 4 hour limit on the calculations in Table 12: beyond this time-limit soldiers may require additional water; in mitigation, however, is the observation that not all hours spent marching would have reached these higher temperatures.
The Work/Rest column for Hard Work in Table 12 shows figures that critically diminish the number of kilometres covered in an hour. Dependent on the WBGT they range from 20 to 50 minutes of rest in each hour; assuming the Roman march velocity was kept constant at 1.2741 m/s (4.59 kph or 2.85 mph) then this would have the effect of increasing the time spent marching the 29 km from 7:22 to 11:11 hours, and with arrival times for the first units stretching from 15:22 to 19:35PM. Crucially, using a 50 minute rest-period, the last unit of the legion would arrive in the camp at 20:59, i.e. after sunset at 20:34.
The figures in Table 12 are described in Bulletin 507 as guidance but, nevertheless, they do have empirical foundations and therefore indicate the physiological/environmental limits that legionaries might have experienced. The WBGT range for the 50 minute rest-period is > 32.22 °C, a value infrequently reached in Britain, however, it should be remembered that the legionary is encased in his 'body armour' and that consequentially an ambient WBGT of only 27 °C requires a compensating adjustment of 5 °C, i.e. close to 32.22 °C. Furthermore, at the other end of the WBGT range, an ambient temperature of only 20 °C, common in Britain, requires an adjustment to 25 °C, and a rest-period of 20 minutes in the hour. What to make of these figures?
First, in mitigation of the stark, rest numbers, the maximum temperatures in Britain are usually limited to three, possible four, hours after midday and, therefore, the occurrence of the more extreme rest-periods would have been limited. Second, it seems highly unlikely, except in the most unusual of circumstances, that legionaries would have been expected to march for over 11 hours to cover the 29 km. Third, the concept of legionaries marching for 10 and then resting for 50 minutes seems untenable. Fourth, these extended hours spent working hard must surely suggest that the legionaries were eating regularly at the rest stops: to do otherwise would have left them without an energy source between extended meal times. This, in turn, suggests that the legionary did carry bread (panis militaris castrensis), or hard tack (hard biscuit, buccelatum), which they consumed at the rest-periods. As an aside, the efficient re-hydration of the human body requires an intake of food with the water; something the Romans may have been aware of.
From these observations, and the consequences of the previously discussed rest-period lengths, it seems reasonable to suppose that the legionary marching in hot weather would either: avoid marching entirely in the hottest hours; decrease his march velocity (which would lower his EE); decrease the distance to be travelled on the hottest days; or combine all three options. It might also be the case that the legionary was trained to only require 10 minutes of rest, or so, even on the hottest days. Whatever options were chosen, or in whatever combination, the overall effect would be to either decrease the distance travelled (except when marching between existing camps or forts), or extend the number of hours spent marching. Presumably the legionary commander defined the pace and destination with regard to the environmental conditions, and also had to ensure that his legionaries arrived at the destination with sufficient energy to build a camp, or engage in some form of military action. Marching between pre-existing temporary marching camps (or forts), as would have been the case in much of southern Britain after the conquest period, would have made legionary life much easier – presuming the pre-existing camp did not require substantial refurbishment. Indeed, travelling between existing camps or forts extends the time available for marching, time otherwise spent building the night-camp. This increased the operational flexibility that the commander could have used on the hottest days. Of course, this flexibility was open only to army units operating in peaceful areas and times, or units re-supplying or reinforcing the 'front line' of a campaign of conquest.
The last point, about marching between existing camps, requires a little explanation. Previously archaeologists had assumed that a marching camp was built, used for one night (maybe 2 or 3), and then abandoned and partially destroyed: hence the term temporary marching camp. This is a generalised description of how it was thought that the camps were used; but, the evidence for destruction seemed to some archaeologists to be at odds with the upstanding remains of many camps in Scotland and elsewhere in highland Britain. However, following more detailed work it is now commonly acknowledged that the camps were not always temporary, they could be re-occupied many times, sometimes extended, sometimes diminished, but rarely (if ever) totally destroyed, at least it seems, not by the Roman army. This is the case in Britain and increasingly being seen to be so in mainland Europe. The word 'temporary' seems to be inappropriate. Therefore, these camps were probably still used by marching legionaries long after their initial building, i.e. into peaceful periods, when their original defensive qualities were no longer paramount. Indeed, the ground on which the original, defensive camp was built would probably become sacrosanct for army use alone, avoided by local farmers and others for centuries in the case of Britain, long after the ditch and rampart had fallen into disrepair for lack of need.
Returning to the issue of energy expenditure (EE) and marching rate we can examine modern studies into the work-rate of soldiers who were allowed to self-pace (work at a self-selected rate, rather than an imposed rate) for extended periods of time. This examination is important because a self-paced rate of work may be close to the natural norm for a large body of soldiers and might represent the sustainable EE level when operating for extended periods. Much of the following is taken from, 'Optimizing Operational Physical Fitness, RTO Technical Report TR-HFM-080. 2009. NATO'. Table 13 shows the self-paced EE and %VO2max for marches over 1, 2.5 and 6 hours.
|
March Duration (h) |
Relative Intensity Self-paced (% VO2max) |
Energy expenditure (kcal/h) |
|
1 |
46 |
549 |
|
2:30 |
40 |
477 |
|
6:30 |
36 |
429 |
Table 13: Self-paced energy expenditure (kcal/h) and % VO2max for marches over 1, 2.5 and 6 hours. Source: Table 3-15, Optimizing Operational Physical Fitness, RTO Technical Report TR-HFM-080. 2009. NATO.
VO2max is the maximal oxygen uptake by volume that can be utilized in one minute during exhaustive exercise. It is the prime factor in determining a soldier's capacity to perform sustained work and is linked to aerobic endurance. It is generally considered the best indicator of cardiorespiratory endurance and aerobic fitness.
Interestingly, training can increase VO2max by up to 20 percent: if legionary recruits were already much fitter and stronger than their modern counterparts, possibly due to their earlier agrarian lifestyle, then they could have more readily reached a high, sustainable rate of work and at a lower percentage of their personal VO2max. If so, they would have had the spare capacity to do more work than their modern counterparts before becoming debilitated by heat stress, etc..
In Table 13 the % VO2max figures decrease from 46% at one hour duration, down to 36 % for 6.5 hours; the corresponding kcal/h rates are 549 and 429, respectively. The referenced NATO report states, “Using a 75 kg man as a model and 36% VO2max as the energy expenditure rate over several days, the average male soldier could perform continuously (with some rest pauses) at an average energy expenditure rate of 429 kcal/h”.
What is particularly significant about the 429 kcal/h figure is its closeness to the figure of 419.68 kcal/h (rest-periods and fatigue at 10% included) for the example legionary (80 kg) marching at 1.2741 m/s (2.85 mph) and carrying a 40 kg load (Table 3) – especially significant when allowing for the NATO soldiers' lower body weight, shorter march duration (6:30 vs 7:22 hours for the legionary) and easier marching surface (black-top).
If the legionary velocity increases to 1.3411 m/s (3.0 mph) the EE rises to 447.04 kcal/h; increase the velocity to 1.609344 m/s (3.6 mph – the British Army's velocity necessary to cover 3 miles in the hour) and the EE rises to 570.64 kcal/h. The latter value exceeds the modern empirical figure (429 kcal/h) by a margin that suggests it is not sustainable over multiple days and would not have been used as a standard by the Roman army (or any other, except for operational necessity). Furthermore, the total, daily EE for the example legionary at this higher velocity rises to 6571 kcal, over 1000 kcal more than when marching at 1.2741 m/s and, as will be discussed later, into an EE range that is only shown by exceptional modern soldiers, conducting extremely vigorous training, and for a short number of hours and days.
Therefore, it seems reasonable to conclude that a march velocity in the range of of 2.85 to 3.0 mph (1.2741 and 1.3411 m/s) was that used by the legionaries on a day-to-day basis, and it is probable that the 2.85 mph figure is nearer the more common velocity.
This 2.85mph velocity was probably more common, at least for traversing a flat road over the 29 km or, more accurately, a road whose slope in aggregate over the 29 km equals 0%, i.e. the road could rise and fall over the 29 km but the sum is 0%. Obviously this is not always going to be the case, as Figure 2 (EE along Roman roads in Britain) demonstrates. Table 14 shows different slope% values over the 29 km distance and the resulting EE for the example legionary conducting all the tasks in Table 3; such roads are typical of those rising from the low-lands onto high ground, e.g. from the Vale of York onto the Pennine ridge, or from the Vale of Evesham, over the Cotswold escarpment and onto the Cotswold plateau, or simply march into Wales!
|
Slope % |
Energy expenditure (kcal per day) |
|
0 |
5504.92 |
|
1 |
5839.11 |
|
3 |
6507.49 |
|
5 |
7175.87 |
|
7 |
7844.24 |
Table 14: Total energy expenditure (kcal per day) for differing slope% values. The example legionary (body weight 80 kg, load 40 kg) conducts all tasks in Table 3. March velocity is 1.2741 m/s. The slope% values are aggregates of all gradients over the 29 km road route.
As a function of the Pandolf equation (Pandolf, 1976), an increase in slope, even 1% or 3%, has a significant effect on the overall energy expenditure of the example legionary; increase the slope to 5% and 7% and the march velocity becomes unsustainable (remember, these are aggregates over the 29 km route). Necessarily, therefore, the Roman unit commander would either decrease the distance to be travelled, lower the velocity and march for longer or, combine the former and latter. The selection would have depended on the terrain and operational need. In this essay these complications of computation due to slope will not be examined any further, not because they are insignificant, but because the effect of slope is localised and best examined along specified routes or roads.
As we have seen, the legionary day was not limited to marching: other strenuous activities (Table 3) were involved which resulted in a total EE that is also instructive, in the sense that, these totals can be compared to modern measurements to determine if there is a natural limit to the daily EE of soldiers working hard, in the field, and for a number of days.
In preparing for later comparisons, Table 15 shows daily EE totals for all the tasks in Table 3 and for legionaries of differing weight (all other parameters kept constant).
|
Legionary body weight (kg) |
Total daily energy expenditure (kcal) |
|
90 |
5693.09 |
|
85 |
5597.22 |
|
80 |
5504.93 |
|
75 |
5417.10 |
|
70 |
5334.90 |
|
65 |
5259.94 |
|
60 |
5194.48 |
Table 15: comparison of total, daily energy expenditure (EE) for legionaries of differing weights. The EE values are generated from the values and calculations shown in Table 3, the schedule of activities for the example legionary. March velocity 1.2741 m/s (2.85 mph, 4.5866 kph). The approx. 500 kcal difference between the 90 and 60 kg legionaries equates to roughly 6 slices (40g) of modern, wholemeal bread (for British readers – Hovis!).
Some modern equivalents to these legionary numbers are shown in Table 16. They originally came from a number of sources, and should be seen as indicative of the empirical measurements taken by military medics/scientists.
|
Population |
Task |
Duration (days) |
Activity ( h/day) |
Energy Expend. (mean kcal/day) |
|
US Marines, mountain warfare |
Ski, snow shoe, bivouac training |
4 |
17.93 |
7131 |
|
Norwegian Ranger Cadets |
Ranger training. Food and sleep deprived |
7 |
21-24 |
6678 |
|
US Marine recruits |
Crucible exercise |
2.25 |
NA |
6129 |
|
Zimbabwean field exercise |
Infantry training |
12 |
8 |
5497 |
|
US Marine officer training |
Combat training |
10 |
18.2 |
5378 |
|
US Army Rangers |
Field training |
8 |
NA |
5185 |
|
US Army Special Forces |
Assessment + training |
20 |
NA |
5182 |
|
All known military studies (pop.424) |
Various |
12.2 (mean) |
Various |
4609 (mean, SD +-645) |
|
Tour de France |
cycling |
22 |
variable |
6066 (mean). 7643 (highest mean, daily) |
Table 16: Examples of energy expenditure for modern soldiers and Tour de France cyclists. All EE values for soldiers calculated using the doubly labelled water method, the most accurate, hence the low total population number (424). Military source: Tharion et al., Energy requirements of military personnel. Appetite 44, 2005. Tour de France source: Saris et al., Study on food intake and energy expenditure during extreme sustained exercise: The Tour de France. Int.J.Sports Med. 10, 1989.
None of the daily activities for the modern military shown in Table 16 can be directly compared to the legionary-day of steady marching, building a camp etc.. However, the prime purpose of Table 16 is to illustrate the upper limits of human endurance and the EE that accompanies it. For example, the EE values for the 'US Marine mountain warfare' and the 'Norwegian Ranger cadets' at 7131 and 6678 kcal/day respectively, are close to sustainability limits and are comparable to legionary EE for aggregate slope% values above 3 (Table 14), i.e. they are extraordinary. After the figures for 'US Marine recruits' (6129 kcal/day) come four groups in the range 5497 to 5182 kcal/day, figures comparable, but lower, to the example legionary at 5504.93 kcal/day.
Therefore, it seems reasonable to suggest, using the parameters already described, that the legionary force was operating at an EE that was exceptional, but not extraordinary.
But how close was the legionary force to EE values that might be classified as extraordinarily unlikely? By simply increasing the march velocity to 1.3411 m/s (3.0 mph, 4.830 kph), and 1.609344 m/s (3.6 mph, 5.79 kph) while keeping all other parameters used in Table 3 constant, causes the EE values to climb to unsustainable levels (Table 17).
|
Legionary body weight (kg) |
Daily energy expenditure (kcal).Velocity 1.2741metres/sec. |
Daily energy expenditure (kcal).Velocity 1.3411metres/sec. |
Daily energy expenditure (kcal).Velocity 1.609344metres/sec. |
|
90 |
5693.09 |
5906.47 |
6870.28 |
|
85 |
5597.22 |
5802.39 |
6729.13 |
|
80 |
5504.93 |
5701.89 |
6591.56 |
|
75 |
5417.10 |
5605.85 |
6458.45 |
|
70 |
5334.90 |
5515.45 |
6330.98 |
|
65 |
5259.94 |
5432.29 |
6210.75 |
|
60 |
5194.48 |
5358.62 |
6100.01 |
Table 17: Comparison of total, daily energy expenditure (EE) for legionaries of differing body weights and for march velocities of 1.2741, 1.3411 and 1.609344 m/s (2.85, 3.0 and 3.6 mph, respectively). Daily tasks itemised in Table 3, all other parameters kept constant.
At a march velocity of 1.3411 m/s (3.0 mph), Table 17 column 3, the EE values are very high, but sustainable. At a velocity of 1.609344 m/s (3.6 mph), column 4, the values are extremely unlikely to be sustainable day-after-day, and can be considered implausible. This latter finding is important because it demonstrates, yet again, that the commonly mentioned, standard, marching pace of the British Army of 3 miles in one hour, together with a 10 minute rest-period, i.e. a march velocity of 3.6 mph, should not be applied to legionaries or British soldiers, except under unusual military circumstances.
At this point it is worth re-emphasising the configuration of the legionary in this study: he carried 40 kg of clothes, armour, arms, personnel equipment and food-stuffs; marched over a flat (0% grade/slope) road a distance of 29 km, and with 10 minute breaks in each hour; and journeyed from one marching camp to a new location, where he built another. Additionally the study is designed to discover, or confirm, variables and factors that would have applied to the normal operation of the Roman Army: hence, examples of extraordinary feats of marching are not of interest.
As we have discussed, the most important variables, other than velocity, in the configuration are load weight and the slope% of the road. The selection of the 40 kg load weight is debatable, others would suggest 35 kg or 43 kg, much of the variation dependent on the weight of the metals used in the arms and armour, and the amount of carried food-stuffs. Not so, however, the effect of slope (Table 14).
If this is increased to a +3% slope for the length of the march then, at 1.2741 m/s, the daily EE rises to 6507 kcal/day for the 80 kg example legionary. A further increase to +5% and the daily EE rises to 7175 kcal/day, a value that just exceeds the 'US Marines Mountain Warfare' values at 7173 kcal/day (Table 16) – extraordinary and unsustainable day-after-day.
In summary, legionaries probably marched on roads at an optimal velocity of between 1.2741 m/s (2.85 mph, 4.5866 kph) and 1.3411 m/s (3.0 mph, 4.828 kph). At a velocity of 1.274 m/s for 29 km, the last ranks of armies greater than 3 legions in size would have arrived after sunset. Velocities above 1.3411 m/s were increasingly stressful, probably not sustainable for long periods (days), and were particularly harsh on the heaviest legionaries. Figure 3 shows arrival times for armies on-road marching at a velocity of 1.274 m/s; it demonstrates that the longer the column, the longer and later the last ranks were marching.
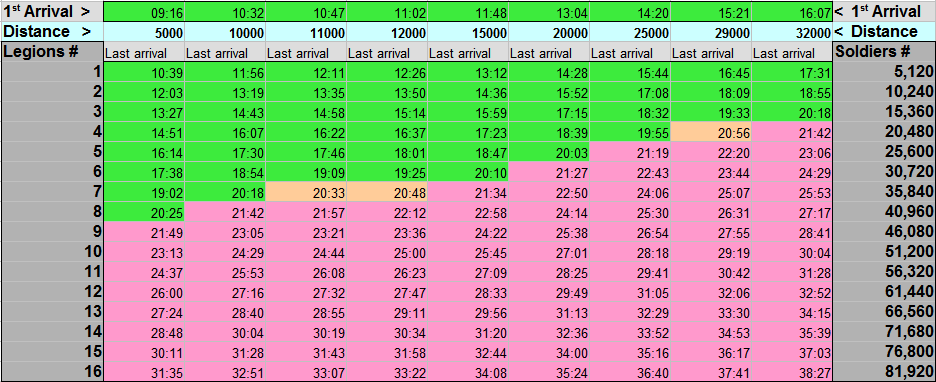
Figure 3: Arrival time matrix for on-road marching at a velocity of 1.2741 m/s (2.85 mph, 4.59 kph) and in a single column. 1st row is the arrival time for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the arrival times for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. The colours green, orange and mauve indicate daylight hours, evening twilight and night-time, respectively. Time is displayed as a continuous clock, i.e. 02:30 h on the second day is displayed as 26:30.
As
previously noted, there is a special case where a road existed
between the marching camps allowing units to conduct a mixture of
off- and on-road marching. The first units would march on-road at a
faster velocity than the second units marching off-road, thus the
first unit out-paced the second, such that eventually the last rank
of the first unit passed the first rank of the second; at this point
the second unit joined the road and accelerated to the faster, road
velocity – and so on for the rest of the army, assuming that
the distance between camps allows the very last unit to join the
road. Clearly, the interplay between the size of armies, differential
velocities, number of gates exited, number of columns and the
distance between camps is complex, and allows for the creation of a
large number of marching configurations and outcomes.
Many archaeologically examined Roman roads have borders, or cleared ground, either side of the road out to 30+ metres (Knapton, 1996); this may have been used by the off-road marching columns in this special case. However, this raises two potential problems: first, the cleared area is often the location of numerous pits where road ballast etc. has been excavated; second, a previously-wooded cleared area would have been littered with tree stumps (assuming the trees were not grubbed-up completely). Both problems suggest that off-road marching in these areas would have been difficult; more agile, and less tightly ranked, columns of horses and pack-mules may have coped, but soldiers marching in tight ranks and carts may have been greatly slowed. Which suggests that off-road columns might have marched beyond the cleared areas. This seems unlikely in Britain, and much of northern Europe, where much of the land beyond the cleared areas might have been wooded, hilly or boggy. Not so for the more open plains of, say, Spain, where soldiers could have marched off-road relatively unencumbered by poor terrain. Therefore, this mix of off- and on-road marching may have been only possible in special circumstances and, probably, when the widely spaced columns were unlikely to be attacked.
Those caveats aside, the method does confer advantages to marching armies, for example the ability to bring the bulk of the army into the new camp earlier than that possible with a single on-road column; a significant drawback was the long, potentially vulnerable tail.
The advantages in time saved were only possible under certain conditions of army size, distance, differential velocities, number of gates exited and number of columns. For example, it might be intuitively expected that a single legion of soldiers, exiting 6 gates and marching 29 km, as one column off- and on-road, would have the last unit arrive in the new camp earlier than the same army using just the road, but, they arrived at 18:08 h compared to 16:46 h; this delay is compounded for all armies (not shown). However, if the number of marching columns was increased to 6 then time was saved, especially for the larger armies (Figure 4).
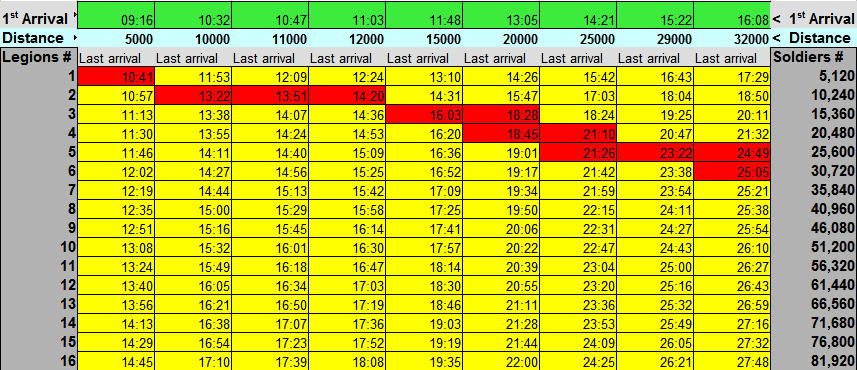
Figure 4: Arrival time matrix for off- and on-road marching using 6 gates and 6 columns. On-road velocity is 1.2741 m/s (2.85 mph, 4.59 kph); off-road velocity 0.6706 m/s (1.5 mph, 2.41 kph). 1st row is the arrival time for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the arrival times for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. The yellow colour denotes an earlier arrival of the last unit compared to the single column, on-road marching shown in Figure 3. The red colour denotes later arrivals. Time is displayed as a continuous clock, i.e. 02:30 h on the second day is displayed as 26:30.
The distribution of the red areas in Figure 4, denoting a later arrival time of the last units compared to the on-road march arrival times in Figure 3, shows how intuition can mislead when considering such complex marching configurations. Indeed, a small loss of velocity, for whatever reason, would have negated the time advantage for smaller armies: as an example, the last unit of a singe legion in Figure 4 arrived at 16:43 h, conferring an advantage of only 2 minutes compared to Figure 3. But, the mathematics gives greater advantages to larger armies, such that, the last unit of a 16 legion army marching 15 km arrived at 19:35 h (Figure 4) versus 34:08 h (Figure 3).
The off-road marching velocity of Roman legionaries.
A search for official, off-road, marching velocities by modern soldiers has revealed only one: 0.6706 m/s (1.5 mph, 2.41 kph) for the US Army (source: Field Manual 21-8, Headquarters, Depart. Of Army, 1990). Other off-road velocities are quoted by various authors, typically pointing out extraordinary efforts due to exceptional operational requirements, but these are not examples of a standard operating procedure.
Other authors quote velocities for modern and Roman soldiers that have been re-cycled from earlier publications and which were themselves of dubious quality. This is understandable because little empirical work has been done on the loaded soldier marching off-road; of course, the modern requirement for rapid and sustained off-road marching has diminished greatly, most off-road activity nowadays being restricted to combat patrolling. An exception occurred during the Falklands War in 1982 when British paratroopers and marines marched for some 90 km across rough terrain. The speed of the traverse is variously given as 3 or 8 days, i.e. 30 or 11.25 km per day; even for a supremely fit and well-motivated soldier a velocity of 30 km/day, across difficult and boggy ground, would be extraordinary and is certainly not sustainable (calculations attest to this statement: marching at 0.6706 m/s, over rough to boggy ground, an 80 kg man carrying a 40 kg load will take 14:29 h to cover 30 km, arriving at 22:29 h having expended 6508 kcal); however, the 11.25 km/day velocity is, as we shall discuss, probably closer to the norm for off-road marching. But, how to confirm this?
Beyond the US Army figure, the earlier description of marching velocities by road gives some possible clues, or limits, to what might have been expected of a legionary marching off-road, i.e. the available time and energy expenditure (EE). Additionally, information from known marching camps may guide the investigation.
The gross time available for all the activity of the marching day is determined by the number of day-light hours. This obvious statement is not meant to imply that night-time marching did not happen, but, if it did, it would probably have been in relatively safe-environments, in the rear of the lead campaigning units, or otherwise done for exceptional operational reasons. To generalise, night-time marching while on campaign in Britain, might have encouraged the local tribesmen to attack the strung-out Roman columns and, hence, is discounted as a possibility.
The next determinant of the available march time
would have been the hours allotted to tasks other than marching (see
Table 3, repeated below): namely, preparing to depart in the morning,
midday meal, building the new camp, erecting the tent, and then
allowing 4 evening hours for washing and cleaning, the main meal of
the day, rest while repairing personal equipment and, finally, 8
hours of sleep. Roughly speaking these other tasks consume 15-16
hours of the day; the remaining 8-9 hours could be spent marching
either on- or off-road.
|
Time (24 hr clock) |
Events |
Legionary activities |
Energy Expenditure kcal/h |
Horse and mule grazing |
Notes |
|
5 |
Morning twilight |
Personal grooming |
176 |
Grazing |
|
|
6 |
Sunrise |
Breakfast. Break camp. |
264 |
|
|
|
7 |
|
Start march |
400.60 |
|
First unit leaves camp |
|
8 |
|
March |
400.60 |
|
Last unit leaves camp 1:23 h after first |
|
9 |
|
March |
400.60 |
|
|
|
10 |
|
March |
400.60 |
|
|
|
11 |
|
Midday meal and break |
132 |
Grazing |
|
|
12 |
|
March |
419.68 |
|
|
|
13 |
|
March |
419.68 |
|
|
|
14 |
|
March |
579.16 |
|
|
|
15:22 |
|
Arrive at new camp. Dig ditch and build rampart |
530 |
Grazing |
Ditch and rampart takes 3-4 hours. |
|
16 |
|
Erect tent |
264 |
Grazing |
Last unit arrives at 16:46 h. |
|
17 |
|
Personal grooming |
176 |
Grazing |
|
|
18 |
|
Evening meal |
132 |
Grazing |
Camp complete. |
|
19 |
|
Rest and repairs |
88 |
Grazing |
|
|
20 |
Sunset |
Rest and repairs |
88 |
Grazing |
|
|
21 |
Evening twilight |
Sleep |
79.25 |
Place animals in camp |
|
|
22 |
|
Sleep |
79.25 |
|
|
|
23 |
|
Sleep |
79.25 |
|
|
|
24 |
Midnight |
Sleep |
79.25 |
|
|
|
1 |
|
Sleep |
79.25 |
|
|
|
2 |
|
Sleep |
79.25 |
|
|
|
3 |
|
Sleep |
79.25 |
|
|
|
4 |
|
Sleep |
79.25 |
|
|
|
|
Total Kilocalories |
|
5504.92 |
|
|
Repeat of Table 3: Typical legionary day. On-road march velocity 1.2741 m/s for one legion. This table is repeated for the ease of the reader. The kcal/h energy expenditure figures are for an 80 kg legionary, marching at 1.2741 m/s (4.59 kph or 2.85 mph), carrying a 40 kg load over a level, Roman road. See the original for further notes.
If we are content that
legionaries typically marched on-road at 1.2741 m/s, could easily
cover 29 km a day, dig a new camp and still have time for 4 evening
hours of grooming, meals and rest, then the hours this took could be
seen as a norm and, if so, the 7:22 to 8:46 h spent marching can be
seen similarly (Table 18, 4th row). Even if the march
distance is increased to 32 km (Table 18, 5th row), a
distance often quoted for modern armies as an upper limit in normal
operations, the time spent marching only increases by approximately
0:45 h, with the last rank arriving at 17:32 h. This last example
does limit the evening task-time from 4:00 to 3:30 h, but this cannot
be seen as critical. It therefore seems reasonable to conclude that
Roman legionaries were expected to march for 7 to 9:30 h each day and
that these times were not exceptional, did not overly tire the
soldiers, and would have been sustainable, that is, the norm for
either on- or off-road marching.
|
Velocity (metres/sec) |
Energy Expenditure (kcal/day) |
Hours marched, first rank ( h:min) |
Hours marched, last rank ( h:min) |
Time of arrival first rank ( h:min) |
Time of arrival last rank ( h:min) |
Distance marched (km) |
|
0.6706 off-road |
4267.44 |
7:14 |
9:54 |
15:14 |
17:54 |
15 |
|
0.7639 off-road |
4438.47 |
7:12 |
9:32 |
15:12 |
17:32 |
17 |
|
|
|
|
|
|
|
|
|
1.2741 on-road |
5504.93 |
7:22 |
8:46 |
15:22 |
16:46 |
29 |
|
1.2741 on-road |
5823.62 |
8:08 |
9:32 |
16:08 |
17:32 |
32 |
Table 18: Figures for off-road marching, single column, by a single legion. See text, above and below, for details. The last 2 rows are benchmarks of the example legionary (Table 3) on-road marching at 1.2741/ m/s (2.85 mph, 4.59 kph) over 29 and 32 km. Off-road velocities of 0.6706 m/s (the US Army off-road rate at 1.5 mph, 2.41 kph) and 0.7639 m/s (1.71 mph, 2.75 kph).
Also in Table 18 figures are shown off-road velocities of 0.6706 m/s (the US Army off-road rate at 1.5 mph, 2.41 kph) and 0.7639 m/s (1.71 mph, 2.75 kph). The last rate is thought to represent a reasonable upper limit to sustained off-road marching in Britain. The figures for march duration have been selected from the calculating spreadsheet to match the norm for hours marched as determined above, i.e. 7:30 to 9:30 h. For the two off-road march velocities, 0.6706 and 0.7639 m/s, the respective times of arrival for the last ranks are 17:54 and 17:32 h, and corresponding distances covered 15 and 17 km. The latest time of arrival decreases the 4 hours of evening time down to approximately 3 hours but this should not be seen as too great a hardship: 3 hours for erecting a tent, personal grooming, eating a meal and then resting while repairing equipment is adequate, especially as each 8 man contubernium had at least one servant/slave assisting. Another servant/slave would have cared for the mule(s), although the late arrival limits the number of hours available to graze the mules and might have meant that the soldiers had to use their scythes to cut fodder – another task to add to the list, although, it is possible that earlier arrivals were tasked with gathering enough fodder for all the animals – such is the way of an army (see Roth, 1998, p.127).
Assuming that the mules marched close to the soldiers, and hence arrived at similar times, then they would have had only 5 hours of grazing time in the day: insufficient to maintain their condition. This supposition further reinforces the idea that mules were, as a norm, tethered and fed fodder as a supplement to grazing.
The energy expenditure for off-road marching (Table 18, 2nd column) is, in both cases, lower than that for the on-road march; this is due to the lower velocity attainable when off-road, i.e. in total, the Roman legionaries would have had to work less hard - they may have preferred marching off-road!
In conclusion to
examining the march velocities, and in reference to Table 18, the
distances covered at the off-road velocities of 0.6706 and 0.7639
m/s, respectively, of 15 and 17 km are relatively easily attained
and, given the variables already discussed, impressively sustainable.
But, this is valid for one legion, less so for 2 legions, and larger
armies would have faced difficulties (Figure 5).
Figure 5: Arrival time matrix for off-road marching at a velocity of 0.6706 m/s (1.5 mph, 2.41 kph) and in a single column. 1st row is the arrival time for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the arrival times for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. The colours green, orange and mauve indicate daylight hours, twilight sunset and night-time, respectively. Time is displayed as a continuous clock, i.e. 02:30 h on the second day is displayed as 26:30.
Figure 5 is a time matrix of arrivals for the first and last ranks with respect to increasing numbers of legions/soldiers; the off-road velocity is 0.6706 m/s and the units are marching in a single column, i.e. this matrix is another method to display the results of the off-road marching we have already discussed. Applying colour to indicate the light status shows that the last rank, of a single legion, and marching for 20 km could have arrived at the new camp at 20:19 h, i.e. the last hour of daylight (orange). However, and crucially, for an army of 2 legions, the last rank would, unacceptably, arrive in the dark (mauve). And, even though it has been demonstrated that 0.6706 m/s is a reasonable march velocity, allowing a traverse of 15 km for a single legion, for a 2 legion army the last rank would arrive in the twilight at 20:33 h. The reason, of course, is due to the army marching in a single column, as if it were marching along a road. To drive the point home, an army of 4 legions, 20,480 soldiers, could not have covered 5 km without the last rank arriving in the dark (Figure 5). Obviously this would not have been acceptable and strongly suggests that multiple-legion armies marched in multiple columns; the detail of this topic will be discussed later.
The energy expenditure for off-road, single column, marching soldiers is displayed in Figure 6. It was shown earlier that, at 0.6706 m/s, a single legion could comfortably and sustainably march 15 km. The EE figures re-emphasize this point: the first arrivals expend only 4267.44 kcal, the last 4231.41 kcal. At 19 km the last rank of a 2 legion army expends 5389.19, still lower than the on-road 'standard' figure of 5504 kcal, but Figure 5 also shows that the soldiers would have arrived after dark at 22:19 h having marched for 12:33 h – dangerous and not sustainable. This example could be replicated for the all the other multiple-legion armies: the longer the column, the longer the last ranks are marching and, hence, the greater the energy expenditure, while the march duration extends into days (for an on-road comparison see Figure 3).
In summary, Figures 5 and 6 in combination show that off-road, single column marching over 15 km or more, and for legion strengths greater than 2, was not a normal, sustainable option and points to other marching strategies. It is often assumed, and written, that Roman legions of a large size simply marched in single column, either off-road or along a road: except for the most exceptional of cases, they normally did not.

Figure 6: The energy expenditure (EE) in kilocalories for soldiers marching off-road in single column. 1st row is the EE for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the EE for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. Velocity 0.6706 m/s; body weight 80 kg; load weight 40 kg; terrain 1.35 (between light and heavy brush). Tasks for the 1st arrivals as in Table 3, i.e. including digging a new camp. Last arrivals do not build the camp. The colours green, orange and mauve indicate kcal values less then 5504, between 5504 and 7000, and greater than 7000, respectively. The 5504 kcal figure is that earlier deemed sustainable; higher kcal expenditures are possible, but the closer to 7000 kcal, the less sustainable is the effort.
Having examined the time available and energy expenditure for off-road marching, we can now turn to the third line of evidence for a common, Roman, off-road velocity: the distances between known temporary marching camps in Britain.
Previous work on known marching camps resulted in Groups of similar size (see: Roman marching camps in Britain: GIS, statistical analysis and hydrological examination of known camp sites, resulting in the prediction of possible camp sites ). The distances between camps in the same Group have been approximately measured and are displayed in Table 19.
|
Group |
Number of legions |
Distance between camps (approx. km) |
Notes |
|
65 to 70 hectares |
9 |
11 - 12 |
3 camps but consistent distance |
|
50 to 60 hectares |
7 - 8 |
10 to 14 |
Poor repetition of distances |
|
40 to 45 hectares |
5 - 6 |
10, 17, 21, 25 |
V. poor repetition of distances |
|
25 hectares |
3 - 4 |
12 to 15 |
Common distances |
|
18 hectares |
2 - 3 |
5 to 10, and 24 |
Poor repetition of distances |
|
13 hectares |
1 |
|
No clear common distance |
Table 19: Common (but approximate) distances between marching camps within Groups.
Figure 7: Arrival time matrix for off-road marching at a velocity of 0.7639 m/s (1.71 mph, 2.75 kph) and in a single column. 1st row is the arrival time for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the arrival times for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. The colours green, orange and mauve indicate daylight hours, twilight sunset and night-time, respectively. Time is displayed as a continuous clock, i.e. 02:30 on the second day is displayed as 26:30.
Note that the distances in Table 19 are approximates, an indication of similar distances. Furthermore, although it is often assumed that camps aligned in series along a road or route might be the result of a single, campaigning army, this is supposition: none have been proven to be linked. That said, some Groups do display a repetition of a common distance(s), for example, Group 65 to 70 hectares and Group 25 hectares. Group 65 to 70 only contains 3 camps, but they are adjacently situated in a line along a road, and are probably the physical manifestation of a very large army of 9+ legions, 46,000 soldiers, advancing northwards into Scottish territory; the common distance is 11 to 12 km. Most camps of Group 25 hectares (3 to 4 legions in size, circa 15,000 to 20,000 soldiers) are located in eastern Scotland, have a distribution that suggests a common provenance or raison d'etre, whatever that may be, and do have a repetition of distances in the range 12 to 15 km. Other Groups do show some commonality from 10 km through to the mid-teens but the repetition of distances in these Groups is less clear. Figure 7 is a time matrix for the faster off-road march velocity of 0.7639 m/s (1.71 mph, 2.75 kph). Cross referencing Table 19 and Figure 7 gives a few insights into how multiple legions might have marched off-road.
First, the army of Group 65 to 70 hectares could not have marched 11 to 12 km between known camps, either on- or off-road, in a single column at 0.7639 m/s: the off-road velocity means that the last rank arrived at the new camp in 33 to 34 h (remember this is timed using a continuous clock). Even marching in single column along a 12 km stretch of road, at 1.2741 m/s, means the last rank arrived at 23:37 h (not shown in tables or figures). Therefore, this army, if it marched as a single unit on one day, must have done so in multiple columns, and this is a valid observation even if one column had use of a road, assuming it existed.
Second, Group 25 hectares, which has common distances between camps of 12 to 15 km, shows that the last rank of a 3-legion-army arrived in the twilight when marching 13 and 14 km, but in the dark at 15 km. The last rank of a 4-legion-army arrived at night for any distance over 9 km. In addition to the danger posed by a lack of daylight, was the crippling effect on the last soldiers of marching for 13 to 15 hours (not shown in tables or figures). As earlier in this section, these examples point to the need for multiple columns to reach the common distances between known marching camps.
Third, Figure 7 shows that only armies of 1 or 2 legions (approx. 5,000 to 10,000 soldiers) could have marched off-road, at this higher velocity, a distance of 15-17 km in daylight, with the last ranks arriving after 11 h of marching (not shown in tables or figures).
In summary of this section, Roman armies of 1 or 2 legions could have sustainably marched off-road, in single column, for 15 or 17 km between camps, at velocities of 0.6706 and 0.7639 m/s, respectively. However, armies greater than 2 legions in size could not have sustainably reached their destination camps without marching in multiple columns (Figure 7). Furthermore, armies of 6 or more legions in size (30,720 soldiers), on-road velocity 1.2741 m/s, could not march more than 10 km without the last ranks arriving after nightfall; to arrive in good time, and in safety, required the legions to march in multiple columns. In conclusion, all armies over 2 legions in size probably marched in multiple columns to reach their destinations.
Roman army multi-column marching.
Findings in previous sections strongly suggest that armies greater than 2 legions of soldiers, 10,240 men, marched in multiple columns, even if a road was present.
A crucial variable that allowed multi-column marching was the many gates constructed in Roman marching camps. Having gates within a ditch and rampart creates points of defensive weakness, nevertheless, multiple gates were routinely built to aid in the collection of forage, fodder and water, and to take the animals to grazing. Having many gates allowed those collecting materials outside the camp to more rapidly find safety if they were attacked while in the open, and the reverse, for rescue-parties to come to their aid. Multiple gates would also have allowed the soldiers to rapidly deploy into the open, and in formation, if they were challenged by another army. Additionally, this author proposes that the main latrine system was external to the camp and linked to the rivers and streams that often partially enclosed the area of a camp (see 'Some comments on the use of rivers.' In: Roman Marching Camps ). Of course, some marching camps were very large, covering many hundreds of metres in length and width, and possibly accommodated circa 62,000 men (soldiers, slaves/servants etc.) which might suggest that they also required many gates to reduce congestion, and spread the erosional foot- and hoof-traffic. For all of these reasons, the larger the army, the greater the number of gates required.
This is confirmed by the archaeological record in Britain: as a generalisation, camps less than about 4 hectares, and typically square, have 4 or fewer gates; those over approximately 4 hectares, and usually with 2 extended sides, have 6 or more gates. An exception is Rey Cross in Scotland which might have had 11 gates (but only 9 are known; Jones, 2011, p.47). Nevertheless, the larger camps in Britain typically have (or are inferred to have) 6 gates – one for each short side and two each on the longer sides. It would be interesting to discover if the width of these gates increases with the size of camp, but this is probably beyond the resolution of the known archaeology.
If multiple gates reduce congestion, erosion and degradation, and allow men to enter and exit at the nearest gate to their tent, then those attributes are clearly beneficial when exiting for the march to a new camp.
Table 20 shows the exit numbers for legions leaving via multiple gates, in this example, soldiers, carts and pack-mules simultaneously use 2 gates apiece. A comparison with legions marching in single column and joining a road is stark, for example Table 20 shows that 1 legion would exit in 00:33 h, whereas the single-column would take 2:39 h (not shown); 16 legions take 8:53 h and the single-column 42:28 (not shown) – both too long, but once again, the detrimental effect of the single-column having to join the road, that acts as a single gate through which all units must pass, is clear.
|
Legions # |
Soldiers exit time (mins) |
Carts exit (mins) |
Pack-mules exit time (mins) |
Total exit time (mins) |
Total time to exit ( h) |
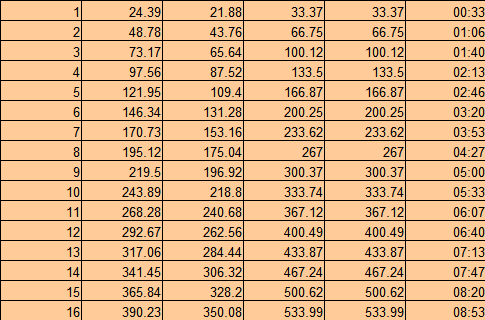
Table 20: Camp exit times for soldiers, carts and pack-mules simultaneously exiting multiple gates. March velocity 0.6706 m/s; simultaneous use of 2 gates for each category.
If the units in Table 20 exit and then join marching columns that match the number of exit gates used by each type, i.e. soldiers, carts and pack-mules each join 2 columns, then no time would be lost in the transition from exiting to marching (Figure 8, Arrival times for units in Table 20). Indeed, if the number of marching columns is greater than the number of exits gates used, then there would have been a saving of time as the total army column length would have been reduced (there is an assumption, that at the point of transition each unit would briefly increase its pace to maintain column integrity). Conversely, if the number of marching columns is less than the exit gates, then the columns are longer, and similarly the travel time. Even in this last, detrimental case, the total time for the march would be less than that of the same unit marching along a road in single column.
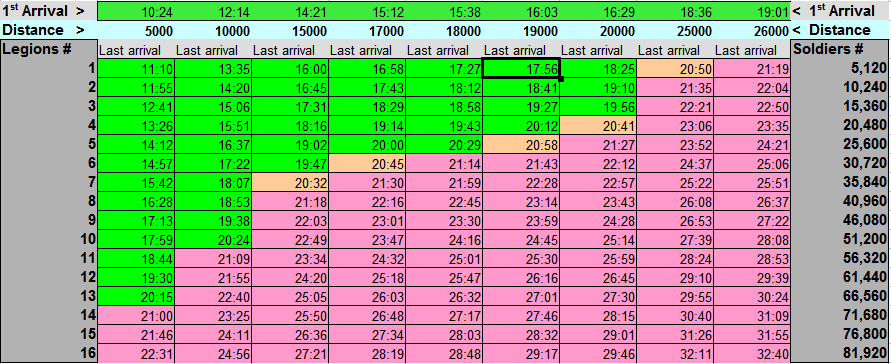
Figure 8: Arrival time matrix for units using the same number of gates and columns. March velocity 0.6706 m/s. Marching columns for soldiers, carts and pack-mules matches simultaneous exit of gates at 2 each. Labels and colours as in Figure 7.
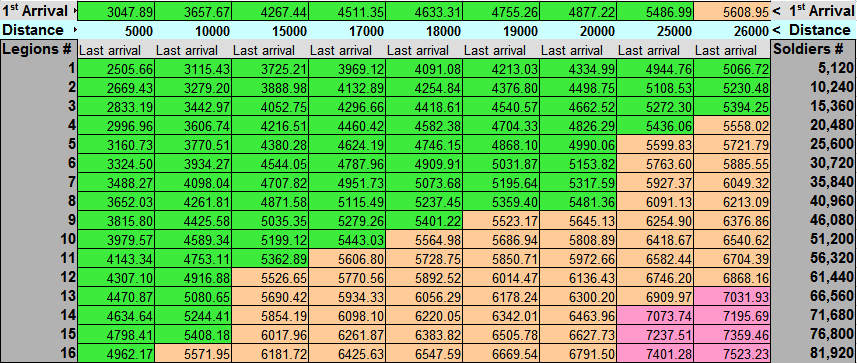
Figure 9: Energy expenditure matrix for units marching in multiple columns as in Figure 8. March velocity 0.6706 m/s. Marching columns for soldiers, carts and pack-mules matches simultaneous exit of gates at 2 each. 1st row is the EE for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the EE for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. Tasks for the 1st arrivals as in Table 3, i.e. including digging a new camp. Last arrivals do not build the camp. The colours green, orange and mauve indicate kcal values less then 5504, between 5504 and 7000, and greater than 7000, respectively. The 5504 kcal figure is that earlier deemed sustainable; higher kcal expenditures are possible, but the closer to 7000 kcal, the less sustainable is the effort.
For Figure 8, the configuration of the marching army is a rectangle made of parallel marching columns, with the cart and pack-mule columns in the centre and flanked either side by columns of soldiers (remember that in this essay only the main body of the army is considered – the reconnaissance, vanguard, command and rear guard units being excluded). Even at this slower, off-road velocity, 0.6706 m/s, a single legion could have covered 20 km by 16:29 h for an EE of 4877.22 kcal (Figure 9) and have the last unit arrive at 18:25 h having expended 4334.99 kcal (the EE for the last units is lower than the first because they are too late to build the camp). Marching units in Group 25 hectare (3 and 4 legions of soldiers), whose common distance between camps is approximately 15 km, may have had an even easier time: the last units arrive between 17:31 h and 18:16 h having expended a maximum of 4216 kcal (Figure 9). Even the Group 65 to 70 hectares (9 legions of soldiers) could have covered their 10 to 11 km common distance by 19:38 h; increase the march velocity to the upper off-road value of 0.7639 m/s and they arrive at 18:13 h for an EE of only 4332 kcal (neither values shown).
The
army of Group 65 to 70 hectares was probably either en-route into or
from Scotland during the Summer time and would not have been
particularly constrained by the number of daylight hours. Not
necessarily so for the units of Group 25 hectares, most of which are
situated in eastern Scotland, and in a manner that suggests that, not
only that they may have been built by units manoeuvring on campaign,
but were also part of a complex interplay between Roman forts and
other 'ground-holding' infrastructure, i.e. the Romans were in
occupation throughout the year (which period(s) is still being
determined). In this case the common distance of 15 km between camps,
and last unit arrival times of 16:21 h to 17:01 h (not shown) at the
faster velocity of 0.7639 m/s, becomes significant when the sunset
time in late December is approximately 15:40 h, that is, the 15 km
common distance might have been set with regard to how far units
could march in daylight to evening twilight in the depths of winter.
For example, a relief-column of one legion of soldiers could have
marched the 15 km with the last unit arriving at 15:01 h -
approximately 40 minutes before sunset on the shortest day. Combining
all known forts and the Group 25 hectare marching camps (Figure 10)
for the area north of the Antonine Wall, also known as the Gask Ridge
frontier system, indicates that the majority of forts lie within a
mutually supporting 15 km radius of each other; where they do not
marching forts are located that bridge the gaps. However, these
supposed gaps (Figure 10: around the yellow vexillation fort and in
the far north) may be due to a lack of archaeological knowledge, i.e.
there may be forts yet to be found. Of course, other factors, such as
the march time for supply trains between forts, would also have
determined the distances between forts and marching camps (see
author's comments in an earlier essay).
Figure 10: Roman auxiliary, vexillation and legionary forts and Group 25 hectare marching camps for the area north of the Antonine Wall, Scotland (The Gask Ridge frontier system). All circles are of radius 15 km suggesting that, in the shortest of winter days, all forts and marching camps could have been reached within day-light hours by a legion of soldiers marching at 0.6706 m/s (1.71 mph, 2.75 kph). The east-west Antonine Wall is marked by the line of dark-green dots. Auxiliary forts are coloured dark-green and with a light-green 15 km buffer; the vexillation fort is yellow; legionary fort is blue; and marching camps are brown spots with a hachured buffer.
Indeed, there are many other factors that determine march distances and times. In all of the calculations the variables used have been those displayed in Table 4 (unless stated otherwise), for example, the pack-mule file number is set to 2 (taken from Peddie, 1994) but, if this is increased to 4, so that each rank of pack-mules is 4 animals wide, then the arrival time of the last units of an army of 9 legions of soldiers, marching at 0.7639 m/s their common distance of approximately 10 km, drops from 18:13 h to 16:36 h (Figure 11). Furthermore, in this configuration the last units could have marched 15 km, arriving at 18:44 h having expended 4538 kcal (not shown), and with neither figure suggestive of a non-sustainable rate. This example is not meant to imply that either a 2 or 4 file arrangement is correct, both are valid, but that when calculating the march times of large armies the smallest of changes in variables can have a dramatic effect; no doubt Roman army commanders were aware of that, and would have altered the configuration of the marching units to suit their needs. Which begs the question, why did this particular army of 9 legions of soldiers only march 10-11 km between camps?: slow, and duration limited, oxen pulling wagons; the driving of cattle to feed the army; or the slow pace of Emperor Septimius Severus and his entourage (it is thought that the Group 65 to 70 hectare camps were the result of the Severan campaign, but no dating evidence exists to support this claim (Jones, 2011, p.48)).
For
armies comparable in size to that at Cannae, i.e. 16 legions of
soldiers (81,920), the simple expedient of increasing the pack-mule
file number to 4 allows the last units to cover 10 km and arrive at
20:00 h, still in daylight and with an EE of 4758 kcal (not shown) –
sustainable but not a great distance. However, there is a method of
exiting the camps, and configuration of marching columns, that
increases the distance covered further still.
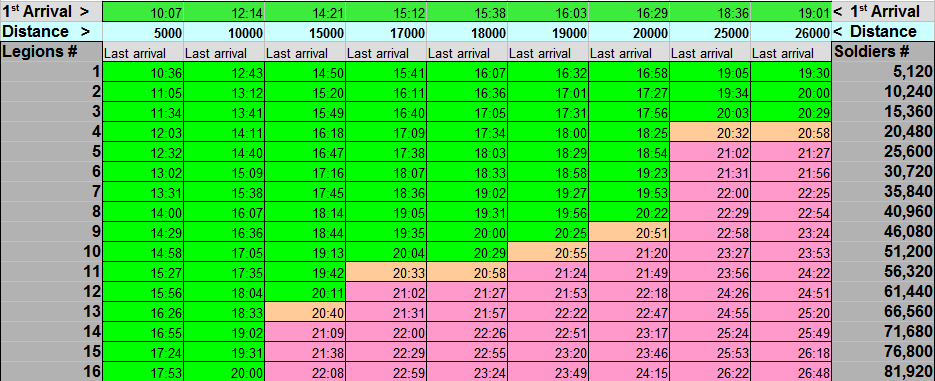
Figure 11: Arrival time matrix for units exiting a camp as in Table 20, but the pack-mule file number has been increased from 2 to 4. Marching columns for soldiers, carts and pack-mules matches simultaneous exit of gates at 2 each. March velocity 0.7639 m/s. Labels and colours as in Figure 4.
This new configuration envisages the use of 8 gates for these larger armies, with each type exiting in series, i.e first, all the soldiers use the 8 gates, followed by all the pack-mules and finally the carts (Table 21). As each type exits it maintains an equality with the number of marching columns, thus, 8 columns of soldiers are in turn followed by 8 columns of pack-mules and, finally, 8 columns of carts. (Note: in this example configuration the pack-mule file number has been returned to the default in Table 4 of 2.)
|
Legions # |
Soldiers exit time (mins) |
Carts exit (mins) |
Pack-mules exit time (mins) |
Total exit time (mins) |
Total time to exit ( h) |
Table 21: Camp exit times for soldiers, carts and pack-mules exiting 8 gates serially. March velocity 0.7639 m/s; all the soldiers use the 8 gates first, followed by all the pack-mules and finally the carts.
In Table 21, the last cart of a 16 legion army will exit the camp 4:39 h after the first soldier and, because of the serial nature of exiting, the pack-mules and carts may have been instructed to only start preparing for the march after, say, half the soldiers had left the camp: this decreased their energy expenditure. The management of this method of exiting was much easier than for mixed units, as in the earlier case, where soldiers, pack-mules and carts used 2 gates each simultaneously. These management and scheduling advantages havenot been modelled.
A possible drawback to exiting and marching serially in blocks is that the pack-mules and carts are to the rear of the soldiers and unprotected by the bulk of the fighting force, indeed, towards the end of the march the last cart is approximately 2:30 h behind the last rank of soldiers; time enough for enemies to ambush, destroy and escape. This disadvantage could be mitigated without slowing the whole army: for example, the block of 8 columns of soldiers exit 8 gates as before, but then the outer four columns are separated into 2 wings of 2 columns, separated by the width of the whole army, and then halted and made to a wait for the block of pack-mules to arrive. At that moment the inner 2 soldier-columns restart their march, but now as flanking units to the block of pack-mules. Similarly, the last 2 soldier-columns wait on the wings until the carts arrive between them and then flank that unit. No time is lost by the army as a whole in this manoeuvring and the pack-mules and carts gain protection. (Note: this description is not meant to imply that the Roman cavalry would not have also provided protection to the marching columns, but flanking legionaries would have been a more robust screen.)
Returning to the
discussion of the simplest case, 8 gates and 8 columns, Figure 12
gives arrival times for the 16 legion army; it could now march 15 km
at 0.7639 m/s with the last cart arriving at 20:42 h. Admittedly,
arriving in twilight was less than ideal, but the figures do indicate
the overall benefit of this exiting and marching configuration. If
the pack-mule file number is again increased from 2 to 4, then the
last arrival time drops to 19:22 h (not shown).
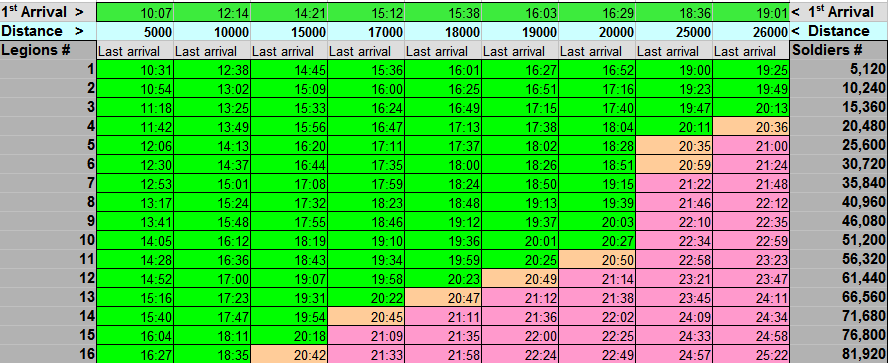
Figure 12: Arrival time matrix for soldiers, carts and pack-mules exiting 8 gates and joining 8 columns. March velocity 0.7639 m/s; all the soldiers use the 8 gates first, followed by all the pack-mules and finally the carts. Labels and colours as in Figure 4.
Another variation in configuration might have been for the 16 legion army of soldiers to split into 2 groups of 8 legions of soldiers, carts and pack-mules. Two separate, 8-legion-groups will collectively take less time to cover the required ground, especially if one group is made to march at a faster pace, arrive at the camp site earlier and build the new camp before the second group arrives.
Which
velocity variation returns the discussion to multiple-column marching
where a road was present, i.e. a mix of off- and on-road marching
(previously discussed in the text around Figure 4). In summary, the
first units march quickly on-road while the second follows more
slowly off-road; once the last ranks of the on-road units have passed
the first rank of the off-road units, then they could have joined the
road and increased their velocity – and so on for following
off-road units. Taking the variables defined in Figure 12 –
off-road velocity 0.7639 m/s, 8 gates and 8 columns – but
having a road available for marching at 1.2741 m/s produces the
arrival time matrix of Figure 13. In this example the last arrivals
of all armies reached their bivouac before their colleagues
multi-column marching off-road (Figure 12). The power of this method
is particularly striking for larger armies, for example, a 16 legion
army, comparable to that at Cannae, completes a 20 km march before
sunset at 19:44h while the off-road equivalent (Figure 12) arrives at
22:49 – long after sunset.
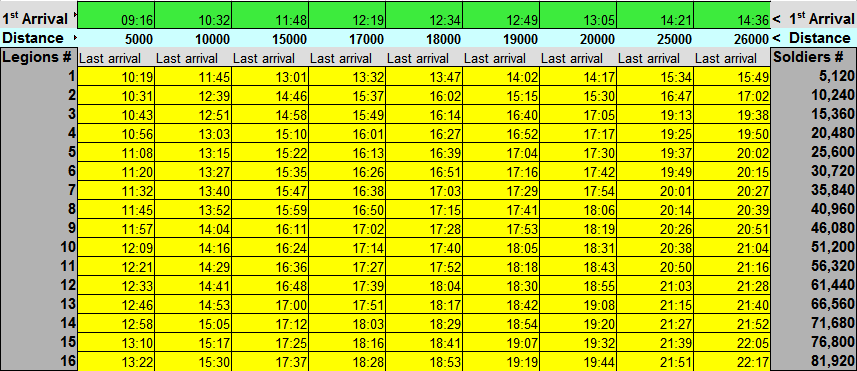
Figure 13: Arrival time matrix for off- and on-road marching using 8 gates and 8 columns. On-road velocity is 1.2741 m/s (2.85 mph, 4.59 kph); off-road velocity 0.7639 m/s (1.71 mph, 2.75 kph). 1st row is the arrival time for the first rank; 2nd row is the distance covered (metres); the columns headed 'Last arrival' contain the arrival times for the last rank with respect to the number of legions and soldiers in far left and right columns, respectively. The yellow colour denotes an earlier arrival of the last unit compared to the multi-column, off-road marching shown in Figure 12. Time is displayed as a continuous clock, i.e. 02:30 h on the second day is displayed as 26:30.
Use of this mixed off- on-road method might have allowed very large armies to move relatively quickly but it was probably only applicable in terrain that was open and free from obstacles, conditions that were relatively rare in northern Europe, and it might be supposed that this method was not commonly employed there.
Summary.
An essay such as this, based almost entirely on the results from parameters in a spreadsheet, ends with much more that could be written. In particular, it could be extended to encompass many more alterations of the parameters in Table 4, e.g. increasing the soldiers file number from 6 to 8, to produce further examples of the marching methods employed by the Romans to project their power, control and influence.
Nevertheless, end it must, and with a list of the main findings (there is no significance in the order):
the Roman legionary probably carried no more than 40 kg of clothing, equipment, food, arms and armour;
a legionary in a typical campaigning day, marching on-road 29 km in approximately 7:30 h and building a temporary marching camp, probably expended between 5500 to 6000 kilocalories of energy, and required the same in replenishment;
following from 2), the legionary would require between 9 and 11 litres of water to avoid dehydration and heat stress;
off-road marching required the expenditure of less energy than when using a road;
a typical legionary (body weight 80 kg, load weight 40 kg, march velocity 1.2741 m/s), could have marched along any of the roads in Britain for an energy expenditure of between of 501 to 542 watts;
Roman legionaries had an on-road march velocity in the range 1.2741 to 1.3411 m/s (2.85 to 3.0mph or 4.59kph to 4.83kph), with the lower value being more likely to have been the more common velocity;
at an on-road velocity of 1.274 m/s for 29 km, the last ranks of armies greater than 3 legions in size would have arrived after sunset, therefore, large Roman armies marched in multiple columns (August 11th daylight hours);
Roman legionaries were expected to march for 7 to 9:30 h each day – these times were not exceptional, did not overly tire the soldiers, and would have been sustainable, that is, the norm for either on- or off-road marching;
off-road velocities were probably in the range 0.6706 m/s (1.5 mph, 2.41 kph) to 0.7639 m/s (1.71 mph, 2.75 kph);
off-road, single column marching over 15 km or more, and for legion strengths greater than 2, was not a normal, sustainable option and requires other marching strategies;
following from 10), all armies over 2 legions in size probably marched off-road in multiple columns to reach their destinations;
a) Roman armies of 1 and 2 legions in size could have marched in single column along a road; b) armies greater than 2, possibly 3, legions marched in multiple columns, whether on- or off-road;
and from the Appendix:
defences for all temporary marching camps, no matter what their perimeter length, might have all been completed in the same amount of time;
following from 13), the defences for a ditch 1.5m wide and 1.0m deep would have been completed in about 2:17 h for all camp sizes;
the largest of defences (greater than width 2.0 m, depth 1.5 m,) might have taken at least a second day to produce;
legionaries in small armies had to work harder than their large-army colleagues.
Finally, many will wonder where the Roman legionary might be listed amongst his modern-day counterparts in terms of marching ability, endurance and sustainable energy expenditure. The short answer is at the very top. A longer answer might would note that the legionary performed on a day-to-day basis, while on campaign or simply travelling across provinces, at a level close to that of the most energetic of modern soldiers – those selected, trained and specialising in extraordinary military activities. The best units of modern armies, for example British paratroopers and marines, could probably be converted to Roman legionary status after suitable training.
Appendix: The building of temporary marching camps.
This appendix deals with some aspects of the variables behind the building of temporary marching camps. Much of what will be discussed is the result of some simple mathematical relationships and the implications that arise.
Many of the variables used here are taken from the work of John Peddie who, being a former British Army officer, had ready access to data collected and collated by his former colleagues (Peddie, 1994 and Table App. 1).
|
Common variables in the digging of a ditch |
|
|
Volume of earth dug by one man (spade). The range representes the difficulty of digging |
0.4 to 0.7 cubic metres/hour |
|
Space occupied by one man digging |
1.524 metres |
Table App. 1: Common variables in the digging of a ditch or trench. Taken from Peddie, 1994. See text for discussion. The 'Volume of earth dug..' variable depends on the difficulty of digging – 0.4 is very hard, 0.7 very easy. It is set to 0.5 cubic metres/hour in the calculations in this essay.
The two critical variables in digging a ditch are shown in Table App. 1: the volume of earth dug by one man in cubic/metre per hour and the space occupied around the camp perimeter by that man. These figures are derived from the experiences of the British Army. The volume removed is determined by the ease with which the ground can be dug. The range is 0.4, for difficult ground, to 0.7 for ground that is easy to dig. The space occupied by a digger is 1.524 metres. These modern variables, of a man using a pickaxe and spade and throwing the soil up to 2 metres away, may not faithfully apply to the Roman legionary.
The legionary was probably using a pickaxe (dolabra) and an entrenching tool (mattock-like), but probably not a spade: the Romans did produce spades, but the blade was made of wood and lined along the periphery with iron; as far as the author knows, no iron-bladed Roman spade has been found. It is not impossible that legionaries used spades for other purposes, but the use of a wooden-bladed device, in previously unbroken ground, seems unlikely. The dolabra had a long wooden shaft, attached to which was the iron-work; one side had the classic pick, the other an elongated blade with the blade edge aligned with the shaft (also possibly for chopping trees, wood and turves). The Roman entrenching tool was similar to the dolabra in general configuration: a long wooden shaft, terminated with iron-work; an elongated blade on one side, and a spade-blade on the other. The modern soldier's spade is designed to be pushed, by either the hands or feet, into the ground and then pick up and throw the soil, but both the Roman devices were designed to be swung by the arms into the ground – to bite into and then drag the released soil from the ditch. The crucial difference between the modern soldier and the legionary, is that the latter probably could not throw the soil; therefore, he dragged the soil behind him where a colleague, possibly with a spade or a basket attached to rope, collected and moved the soil up onto the rampart where a further colleague tamped. In terms of energy expenditure, the modern soldier's work might have been shared by two Roman legionaries – the digger and collector; this probably resulted in slightly faster digging and rampart building, and would have eased the daily work-load, and lowered the EE.
If this hypothesis is correct, then the total team of legionaries in each 1.541 metre section around the camp periphery might have been four: one digging; one transporting the spoil by spade or basket up to the rampart; one receiving the spoil on the rampart; and one tamping the rampart. Any more than four men would result in over-crowding, any less and the soil transport and tamping is less efficient. And, of course, each legionary could have spent time digging in his turn thereby sharing the work-load and energy expenditure of digging.
As an aside, one can envisage a contubernium (a group of eight legionaries who shared a tent) arriving at the location for their tent; four men dropped their personal equipment, arms and armour and marched off to dig and build the rampart. They already knew exactly where to go because each section of the ditch/rampart was linked to the location of the tent. The other four raised the tent and otherwise prepared the site for the evening and night. Once that was completed, say after an hour, they relieved their comrades digging the ditch and rampart; both four man digging teams then shared the task in turn until completion, at which point the day's hard labour is over and they can all clean themselves at the nearest river or stream (17:00 h, Table 3, main text). This task-sharing among eight-man-teams means that, if the defences took 2 hours to build, then each man need only dig the ditch, normally the most energy intensive activity of the day, for 15 minutes; if the defences took 3 hours to build, then the digging time only increases to 22 minutes. Speculation, of course, but there is some logistical logic in such a scheme.
Returning to the main narrative, and leaving aside the possible differences in equipment, together with the consequences that flow from that, the modern, variable values (Table App. 1) will be maintained in this essay, even though the legionary method may have been more efficient.
It seems reasonable to assume that digging the ditch took the most time when producing the defences of a marching camp (Figure App. 1). Although the final work preparing the rampart, for example lining it with turves, or setting stakes or caltrops, might have been the last building acts, they would have had to wait until the ditch was completed; in any case, with many hands available, they would only take a few minutes effort, which isnot thought to be significant when calculating the total time taken to produce the defences.

Figure App. 1: Digging variables and times for three, differently sized, legionary marching camps. The example camps are: Cogar Green (the orange block), one legion (5120) sized body of soldiers; Raedykes (green block), approx. five legions; and St. Leonard's Hill (blue block, approx. Nine legions of soldiers. The perimeter values are taken from the archaeological record and the number of diggers calculated according to the space required for each digger (Table App. 1). The width and depth (metres) numbers in the 'Ditch Size' (yellow) rows set the base for the volume (cubic metre), man-hours and hours to dig figures for each camp size. The variable for the difficulty of digging is set to 0.5 cubic metres/soldier/hour. The volume is calculated for a triangular prism – 0.5 x breadth x height x length. The number of soldiers contained in the camps is set by a density of 690 per hectare.
Figure App.1 shows very clearly the effect of having the same amount of space available for each digging soldier, namely 1.524 metres, irrespective of the length of the perimeter: defences for all camps, no matter what their perimeter length, might have all been completed in the same amount of time. As an example, the defences for a ditch 1.5 m wide, 1.0 m deep and a top-of-the-rampart to bottom-of-the-ditch height (henceforth called the 'throw') of approximately 2 m (excluding any form of palisade), would have been completed in about 2:17 h for all camp sizes. If the need for defence was urgent then more men could have been squeezed into the perimeter by shrinking the digging-space requirement to, say, 1.25 metres. But shrinking that space any further, for a man wielding a pick and entrenching tool, would have been difficult and dangerous.
At 1.524 metres spacing,
all camps could produce defences with a throw of approximately 3
metres in 4:34 h; a time that suggests a limit for a single day,
unless the need was so great that night-time digging was sanctioned.
In turn this might mean that the largest of defences (greater than
width 2.0 m, depth 1.5 m, Figure App. 1) would have taken at least a
second day to produce. This observation might be echoed in the
archaeological record where ditches are commonly recorded between 0.8
and 1.5 metres in depth (Gilliver, 1993). However, the variable for
the difficulty of digging, represented by the selected value of 0.5
cubic metres/soldier/hour, is at the more difficult end of the range,
0.4 to 0.7, and chosen to represent the ground commonly found in
northern Britain. Lighter soil would have been removed more quickly,
thereby speeding the process of building the camp. This interplay,
between the time to complete the defences and the ground difficulty,
might be reflected in the varying depths of ditches around some of
the known camps in Britain. For example, the western side of Cogar
Green (Figure App. 1) is underlain by clay soil and the ditch is
small, whereas the sandy, north eastern side is wide and deep
(Gilliver, 1993). It might be argued that in this example, and many
others in Britain, that the time available for digging at the end of
the marching-day was the controlling parameter and, furthermore, if a
known camp shows such features then they may be indicators of camps
only occupied for a single night. Of course, the camp might have been
otherwise secure, e.g. in friendly territory where it was not thought
necessary to extend the ditches on later days.
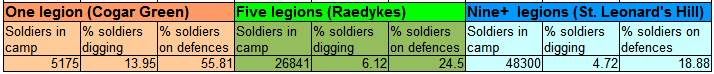
Figure App. 2: Some percentages for soldiers digging ditches and building the defences. The chosen camps, and associated colours, are the same as those in Figure App. 1. The percentages are based on a four man team, with one digging. Hence, for Cogar Green, the diggers represent 13.95% of all soldiers in the camp, while the four-man-team building the defences represents 55.81%.
The mathematics of perimeters and areas enclosed, together with the space required by each digger, determines the percentages of diggers, and those building the defences (four-man-team), relative to the total number of soldiers in the camp (Figure App. 2). For smaller camps the percentage of soldiers building the defences must always be greater than those for larger camps, hence, for a one legion sized army 55.81% of all soldiers would be engaged in defence-building at any one time, but the figure is only 18.88% for a nine+ legion army. This has some interesting implications.
First, individual soldiers in small armies were more likely to have to engage in defence-building, and will expend more energy and consume more food as a result. Couple this with the greater likely-hood of smaller armies marching on-road, if one existed, and hence expending more energy than their larger, multi-column, off-road marching colleagues (see the main text), and it seems likely that the relative cost of operations was greater for small armies, than for large: simply, the soldiers of the smaller armies required more food.
Second, the ancient accounts relate events when Roman armies were attacked as they built the defences. It is for this reason that, we are told, part of the army stood on guard while their colleagues built. Assuming that the builders were organised into half a contubernium (four-man-teams), then for a single legion army with 55.81% of all men engaged on defences, the number of soldiers available for guard duty is limited, even more so if other camp tasks are included. The overall result is that, relative to the larger armies, more men are working for longer hours. In addition, as Figure App. 2 shows, the men available for guard duty would have been less than those engaged on the defences: therefore, it may have been more dangerous to be building a small camp, than a larger one.
If the hypothesis that eight-man-teams shared the building of defences is considered, then the percentages, of course, double. Now all soldiers in a single legion army were engaged in building; none, or at least very few, were available for force-protection. For a mixed legionary-auxiliary force this raises the possibility that infantry auxiliaries were also engaged in camp building. These situationswere probably avoided by some other allotment of task-sharing – one can devise a number of possibilities, each plausible, but none which could be substantiated in the archaeological record. Nevertheless, if eight-man-teams were standard, then the earlier observation that men in smaller armies had to work harder, and in more dangerous situations than their large-army colleagues, is further supported.
Bibliography.
Gilliver, C.M. 1993. The Roman Art of War: theory and practice (Ph.D. thesis). Institute of Archaeology, University College, London.
Jones, R.H. 2011. Roman Camps in Scotland. Society of Antiquaries of Scotland.
Knapton, J. 1996. The Romans and their roads – the original small element pavement technologists. Proc. 5Th Int. Conf. On Concrete Block Paving. Pp. 16-52. Pave Israel '96. Dan Knassim Ltd., Tel Aviv.
Pandolf, K.B., et. al. 1976. Predicting energy expenditure with loads while standing or walking very slowly. US Army Research Institute of Environmental Medicine, Natick. Massachusetts.
Peddie, J. 1994. The Roman War Machine. Alan Sutton Publishing Ltd.
Roth, J.P. 1998. The Logistics of the Roman Army at War (264 B.C. - A.D.235). Brill.
Saris, W.H.M., et al. 1989. Study on food intake and energy expenditure during extreme sustained exercise: The Tour de France. Int.J.Sports Med. 10.
Tharion, W.J., et al. 2005. Energy requirements of military personnel. Appetite 44, 47-65.
Whipp, B.J., et. al. 1998. Paleo-bioenergetics: the metabolic rate of marching Roman legionaries. Br J Sports Med. 32(3): 261–262.
Copyright.
This essay is Copyright © Steve Kaye and protected under UK and international law. May be used free of charge. Selling without prior written consent prohibited. Obtain permission before redistributing. In all cases this notice must remain intact.
Elements within this essay, specifically the original marching camp data and Roman roads, are © Crown Copyright. All rights reserved 2013. This copyright is acknowledged for all images and text in which such elements reside.